Advertisements
Advertisements
प्रश्न
The curved surface area of a right circular cone of height 15 cm and base diameter 16 cm is
विकल्प
60π cm2
68π cm2
120π cm2
136π cm2
उत्तर
136 cm2
Explanation;
Hint:
Here, h = 15 cm, r = 8 cm
l = `sqrt("h"^2 + "r"^2)`
= `sqrt(15^2 + 8^2)`
= `sqrt(225 + 64)`
= `sqrt(289)`
= 17
C.S.A of a cone
= πrl sq.units.
= π × 8 × 17
= 136π cm2
APPEARS IN
संबंधित प्रश्न
Find the ratio of the curved surface areas of two cones if their diameters of the bases are equal and slant heights are in the ratio 4 : 3.
The circumference of the base of a 10 m height conical tent is 44 metres. Calculate the length of canvas used in making the tent if width of canvas is 2 m. (Use it 𝜋= 22/7).
The radius and the height of a right circular cone are in the ratio 5 : 12. If its volume is 314 cubic meter, find the slant height and the radius (Use it 𝜋 = 3.14).
The radius and height of a right circular cone are in the ratio 5 : 12 and its volume is 2512 cubic cm. Find the slant height and radius of the cone. (Use it 𝜋 = 3.14).
The circumference of the base of a 12 m high conical tent is 66 m. Find the volume of the air contained in it.
A solid metal sphere is cut through its center into 2 equal parts. If the diameter of the sphere is `3 1/2 cm`, find the total surface area of each part correct to two decimal places.
A solid cone of radius 5 cm and height 8 cm is melted and made into small spheres of radius 0.5 cm. Find the number of spheres formed.
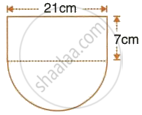
The given figure shows the cross section of a water channel consisting of a rectangle and a semi-circle. Assuming that the channel is always full, find the volume of water discharged through it in one minute if water is flowing at the rate of 20 cm per second. Give your answer in cubic metres correct to one place of decimal.
A solid is in the form of a right circular cone mounted on a hemisphere. The diameter of the base of the cone, which exactly coincides with hemisphere, is 7 cm and its height is 8 cm. The solid is placed in a cylindrical vessel of internal radius 7 cm and height 10 cm. How much water, in cm3, will be required to fill the vessel completely?
Surface area of a cone is 188.4 sq.cm and its slant height is 10 cm. Find its perpendicular height ( π= 3.14)
