Advertisements
Advertisements
प्रश्न
Surface area of a cone is 188.4 sq.cm and its slant height is 10 cm. Find its perpendicular height ( π= 3.14)
उत्तर
Let the radius of the base and perpendicular height of the cone be r cm and h cm, respectively.
Slant height of the cone, l = 10 cm
Surface area of the cone = 188.4 cm2
∴ πrl = 188.4 cm2
⇒ 3.14 x r x 10 = 188.4
⇒ r = `[188.4]/[3.14 xx 10]` = 6 cm
Now,
r2 + h2 = l2
⇒ (6)2 + h2 = (10)2
⇒ 36 + h2 = 100
⇒ h2 = 100 - 36 = 64
⇒ h = `sqrt 64` = 8 cm.
Thus, the perpendicular height of the cone is 8 cm.
APPEARS IN
संबंधित प्रश्न
The radius of a cone is 5 cm and vertical height is 12 cm. Find the area of the curved surface.
Find the ratio of the curved surface areas of two cones if their diameters of the bases are equal and slant heights are in the ratio 4 : 3.
A right angled triangle of which the sides containing he right angle are 6.3 cm and lo cm in length, is made to turn round on the longer side. Find the volume of the solid, thus generated. Also, find its curved surface area.
The curved surface area of a cone is 12320 cm2. If the radius of its base is 56 cm, find its height.
The radius and the height of a right circular cone are in the ratio 5 : 12 and its volume is 2512 cubic cm. Find the radius and slant height of the cone. (Take π = 3.14)
The diameter of two cones are equal. If their slant heights are in the ratio 5 : 4, find the ratio of their curved surface areas.
A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water up to the brim. When lead shots, each of which is a sphere of radius 0.5 cm, are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped in the vessel.
A sphere and a cone have the same radii. If their volumes are also equal, prove that the height of the cone is twice its radius.
Find the cost of painting a hemispherical dome of diameter 10 m at the rate of Rs 1.40 per square metre.
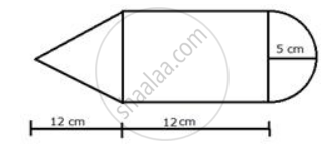
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the total volume of the solid.