Advertisements
Advertisements
प्रश्न
When a resistance of 3Ω is connected across a cell, the current flowing is 0.5 A. On changing the resistance to 7Ω, the current becomes 0.25A. Calculate the e.m.f. and the internal resistance of the cell.
उत्तर
I = `"E"/("R + r")`
∴ `0.5 = "E"/(3 + "r")` ....(as I = 0.5 A when R = 3Ω)
∴ E = (3 + r) × 0.5 ....(i)
also 0.25 = `"E"/(7 + "r")` ...(as I = 0.25 A when R = 7 W)
∴ E = (7 + r) × 0.25 ....(ii)
Comparing equation (i) and (ii)
E = (3 + r) × 0.5
= (7 + r) × 0.25
∴ 1.5 + 0.5r = 1.75 + 0.25r
∴ 0.25 r = 0.25
Internal resistance = r = 1 Ω
Now E = I (R + r)
∴ E = 0.5(3 + 1) (as I = 0.5 A when R = 3 Ω, r = 1 Ω)
∴ E = 0.5 + 1.5 = 2 V
E.m.f. of cell E = 2 volt = 2 V.
APPEARS IN
संबंधित प्रश्न
A cell of Emf 2 V and internal resistance 1.2 Ω is connected with an ammeter of resistance 0.8 Ω and two resistors of 4.5 Ω and 9 Ω as shown in the diagram below:

1) What would be the reading on the Ammeter?
2) What is the potential difference across the terminals of the cell?
Name two factors on which the internal resistance of a cell depends and state how does it depend on the factors stated by you.
A cell of e.m.f ε and internal resistance r is used to send current to an external resistance R. Write expressions for
- the total resistance of circuit.
- the current drawn from the cell.
- the p.d. across the cell.
- voltage drop inside the cell.
A battery of e.m.f 3.0 V supplies current through a circuit in which the resistance can be changed.
A high resistance voltmeter is connected across the battery. When the current is 1.5 A, the voltmeter reads 2.7 V. Find the internal resistance of the battery.
A cell of e.m.f. 1.8V and internal resistance 2Ω is connected in series with an ammeter of resistance 0.7Ω and a resistor of 4.5Ω as shown in Fig.
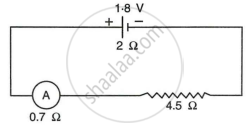
- What would be the reading of the ammeter?
- What is the potential difference across the terminals of the cell?
A battery of e.m.f. 15 V and internal resistance 3 ohm is connected to two resistors of resistances 3 ohm and 6 ohm is series Find:
(a) the current through the battery
(b) the p.d. between the terminals of the battery.
A cell of e.m.f. ε and internal resistance 𝔯 sends current 1.0 A when it is connected to an external resistance 1.9 Ω. But it sends current 0.5 A when it is connected to an external resistance 3.9 Ω. Calculate the values of ε and 𝔯.
Define the e.m.f. (E) of a cell and the potential difference (V) of a resistor R in terms of the work done in moving a unit charge. State the relation between these two works and the work done in moving a unit charge through a cell connected across the resistor. Take the internal resistance of the cell as ‘r’. Hence obtain an expression for the current i in the circuit.
Four cells each of e.m.f. 2V and internal resistance 0.1 Ω are connected in series to an ammeter of negligible resistance, a 1.6 Ω resistor and an unknown resistor R1. The current in the circuit is 2A. Draw a labelled diagram and calculate:
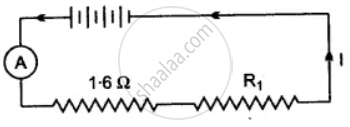
(i) Total resistance of the circuit,
(ii) Total e.m.f.
(iii) The value of R1 and
(iv) The p.d. across R1.
(a) Calculate the total resistance across AB.
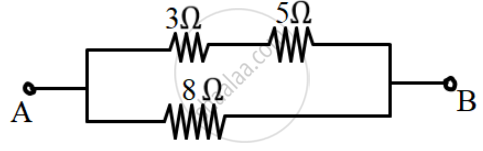
(b) If a cell of e.m.f 2.4 V with negligible internal resistance is connected across AB then calculate the current drawn from the cell.
