Advertisements
Advertisements
प्रश्न
Which point on the y-axis is equidistant from (2, 3) and (−4, 1)?
उत्तर
The distance d between two points `(x_1,y_1)` and `(x_2,y_2)` is given by the formula
`d = sqrt((x_1 - x_2)^2 + (y_1 - y_2)^2)`
Here we are to find out a point on the y-axis which is equidistant from both the points A (2, 3) and B (−4, 1).
Let this point be denoted as C(x, y).
Since the point lies on the y-axis the value of its ordinate will be 0. Or in other words, we have x = 0.
Now let us find out the distances from ‘A’ and ‘B’ to ‘C’
`AC = sqrt((2 - x)^2 + (3 - y)^2)`
`= sqrt((2 - 0)^2 + (3 - y)^2)`
`AC = sqrt((2)^2 + (3 - y)^2)`
`BC = sqrt((-4-x)^2 + (1 - y)^2)`
`= sqrt((-4-0)^2 + (1 - y)^2)`
`BC = sqrt((-4)^2 + (1 - y)^2)`
We know that both these distances are the same. So equating both these we get,
AC = BC
`sqrt((2)^2 + (3 - y)^2) = sqrt((-4)^2 + (1 - y)^2)`
`(2)^2 + (3 - y)^2 = (-4)^2 + (1 - y)^2`
`4 + 9 + y^2 - 6y = 16 + 1 + y^2 - 2y`
4y = -4
y = -1
Hence the point on the y-axis which lies at equal distances from the mentioned points is (0, -1)
APPEARS IN
संबंधित प्रश्न
Name the quadrilateral formed, if any, by the following points, and given reasons for your answers:
A(4, 5) B(7, 6), C (4, 3), D(1, 2)
Show that the points A (1, 0), B (5, 3), C (2, 7) and D (−2, 4) are the vertices of a parallelogram.
Show that the points A(6,1), B(8,2), C(9,4) and D(7,3) are the vertices of a rhombus. Find its area.
Points P, Q, and R in that order are dividing line segment joining A (1,6) and B(5, -2) in four equal parts. Find the coordinates of P, Q and R.
In what ratio is the line segment joining A(2, -3) and B(5, 6) divide by the x-axis? Also, find the coordinates of the pint of division.
Find the area of quadrilateral ABCD whose vertices are A(-3, -1), B(-2,-4) C(4,-1) and D(3,4)
If the point P(k-1, 2) is equidistant from the points A(3,k) and B(k,5), find the value of k.
If A(3, y) is equidistant from points P(8, −3) and Q(7, 6), find the value of y and find the distance AQ.
\[A\left( 6, 1 \right) , B(8, 2) \text{ and } C(9, 4)\] are three vertices of a parallelogram ABCD . If E is the mid-point of DC , find the area of \[∆\] ADE.
If the centroid of the triangle formed by points P (a, b), Q(b, c) and R (c, a) is at the origin, what is the value of a + b + c?
If (x , 2), (−3, −4) and (7, −5) are collinear, then x =
If points (a, 0), (0, b) and (1, 1) are collinear, then \[\frac{1}{a} + \frac{1}{b} =\]
If Points (1, 2) (−5, 6) and (a, −2) are collinear, then a =
Any point on the line y = x is of the form ______.
If segment AB is parallel Y-axis and coordinates of A are (1, 3), then the coordinates of B are ______
Points (1, –1) and (–1, 1) lie in the same quadrant.
Find the coordinates of the point whose ordinate is – 4 and which lies on y-axis.
In which quadrant, does the abscissa, and ordinate of a point have the same sign?
The distance of the point (–4, 3) from y-axis is ______.
Ryan, from a very young age, was fascinated by the twinkling of stars and the vastness of space. He always dreamt of becoming an astronaut one day. So, he started to sketch his own rocket designs on the graph sheet. One such design is given below :
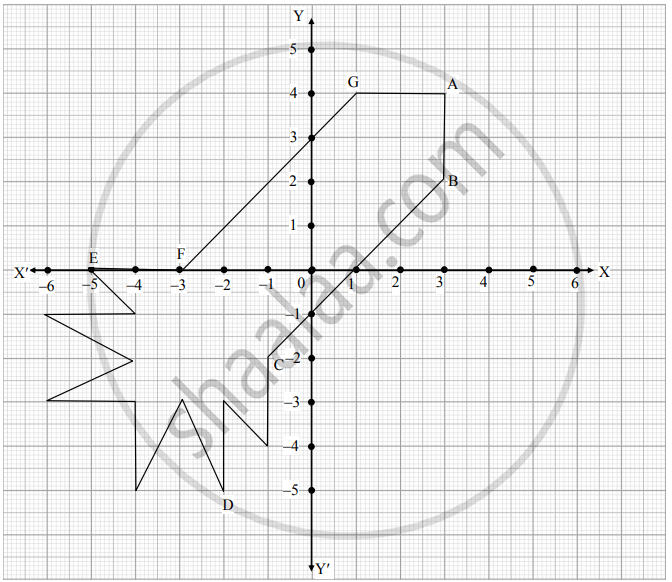
Based on the above, answer the following questions:
i. Find the mid-point of the segment joining F and G. (1)
ii. a. What is the distance between the points A and C? (2)
OR
b. Find the coordinates of the points which divides the line segment joining the points A and B in the ratio 1 : 3 internally. (2)
iii. What are the coordinates of the point D? (1)
