Advertisements
Advertisements
प्रश्न
`2sqrt2a^3 + 16sqrt2b^3 + c^3 - 12abc`
उत्तर
`= (sqrt2a)^3 + (2sqrt2b)^3 + c^3 - 3 xx sqrt2a xx 2sqrt2b xx c`
`= (sqrt2a _ 2sqrt2b + c)((sqrt2a)^2 + (2sqrt2b)^2 + c^2 - (sqrt2a)(2sqrt2b) - (2sqrt2b)c - (sqrt2a)c)`
`= (sqrt2a + 2sqrtb + c)(2a^2 + 8b^2 + c^2 - 4ab - 2sqrt2bc - sqrt2ac)`
`2sqrt2a^3 + 16sqrt2b^3 + c^3 - 12abc = (sqrt2a + 2sqrt2b + c)(2a^2 + 8b^2 + c^2 - 4ab - 2sqrt2bc - sqrt2ac)`
APPEARS IN
संबंधित प्रश्न
Get the algebraic expression in the following case using variables, constants and arithmetic operations.
Number 5 added to three times the product of numbers m and n.
Factorize `2a^2 + 2 sqrt6ab + 3b^2`
Factorize: a2 + b2 + 2 (ab + bc + ca)
Factorize x4 + x2 y2 + y4
Find the value of the following expression: 81x2 + 16y2 − 72xy, when \[x = \frac{2}{3}\] and \[y = \frac{3}{4}\]
Simplify : \[\frac{1 . 2 \times 1 . 2 \times 1 . 2 - 0 . 2 \times 0 . 2 \times 0 . 2}{1 . 2 \times 1 . 2 + 1 . 2 \times 0 . 2 + 0 . 2 \times 0 . 2}\]
Evaluate:
(i) (a + b)(a - b)
(ii) (a2 + b2)(a + b)(a - b); using the result of (i).
(iii) (a4 + b4)(a2 + b2)(a + b)(a - b); using the result of (ii).
Express the following as an algebraic expression:
The product of 12, x, y and z minus the product of 5, m and n.
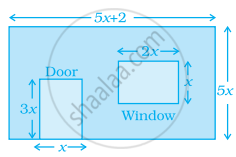
The figure shows the dimensions of a wall having a window and a door of a room. Write an algebraic expression for the area of the wall to be painted.
In the expression 2πr, the algebraic variable is ______.
