Advertisements
Advertisements
प्रश्न
A calf is tied with a rope of length 6 m at the corner of a square grassy lawn of side 20 m . If the length of the rope is increased by 5.5 m , find the increase in area of the grassy lawn in which the calf can graze .
उत्तर
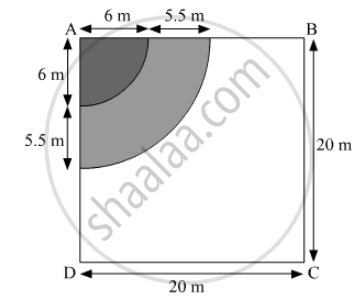
The area grazed by the calf is in the form of a quadrant of a circle with radius 6 m.
Area grazed by the calf with rope 6 m=`1/4pi(6)^2`
`=28.28 m^2`
When the rope length is increased then total rope length = 6 + 5.5 m = 11.5 m
Area covered by the calf for grazing with rope `11.5 m=1/4pi(11.5)^2`
`=103.91 m^2`
Hence, increase in area of grassy lawn that is grazed = 103.91 − 28.28 = 75.63 m2
APPEARS IN
संबंधित प्रश्न
A circle of radius 2 cm is cut out from a square piece of an aluminium sheet of side 6 cm. What is the area of the left over aluminium sheet? (Take π = 3.14)
A 36-m-long, 15-m-borad verandah is to be paved with stones, each measuring 6dm by 5 dm. How many stones will be required?
In the following figure, ABCD is a trapezium of area 24.5 cm2 , If AD || BC, ∠DAB = 90°, AD = 10 cm, BC = 4 cm and ABE is quadrant of a circle, then find the area of the shaded region. [CBSE 2014]

What is the ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal?
Find the ratio of the area of the circle circumscribing a square to the area of the circle inscribed in the square .
If diameter of a circle is increased by 40%, then its area increase by
The cost of fencing a circular field at the rate of Rs 25 per metre is Rs 5500. The field is to be ploughed at the rate of 50 paise per m2 . Find the cost of ploughing the field. [Take `π =22/7`].
The diameters of two circles are 32 cm and 24 cm. Find the radius of the circle having its area equal to the sum of the areas of the two given circles.
Thenmozhi wants to level her circular flower garden whose diameter is 49 m at the rate of ₹ 150 per m2 Find the cost of levelling
The area of a circle is 154 cm2. Its diameter is ____________.
