Advertisements
Advertisements
प्रश्न
A circle of maximum possible size is cut from a square sheet of board. Subsequently, a square of maximum possible size is cut from the resultant circle. What will be the area of the final square?
पर्याय
`3/4` of original square.
`1/2` of original square.
`1/4` of original square.
`2/3` of original square.
उत्तर
`bb(1/2 "of original square")`.
Explanation:
Let a be the side of a square sheet.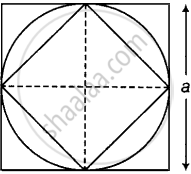
Then, area of bigger square sheet = a2 ...(i)
Now, we make the circle of maximum possible size from it.
Then, the radius of circle = `a/2` ...(ii)
So, its diameter (d) = `2 * a/2 = a`
Now, any square in a circle of maximum size will have the length of diagonal equal to the diameter of circle.
i.e Diagonal of square made inside the circle = a
So, the side of this square = `a/sqrt(2)` ...[∵ Diagonal = side `sqrt(2)`]
∴ Area of this square = `a^2/2` ...(iii)
From equations (i) and (iii),
Area of final square is `1/2` of original square.
APPEARS IN
संबंधित प्रश्न
The `3/4` th part of a conical vessel of internal radius 5 cm and height 24 cm is full of water. The water is emptied into a cylindrical vessel with internal radius 10 cm. Find the height of water in cylindrical vessel.
A tank of cylindrical shape has radius 2.8 m and its height 3.5 m. Complete the activity to find how many litres of water the tank will contain.
Capacity of water tank = Volume of cylindrical tank
= πr2h
A spherical shell of lead, whose external diameter is 18 cm, is melted and recast into a right circular cylinder, whose height is 8 cm and diameter 12 cm. Determine the internal diameter of the shell.
Height of a solid cylinder is 10 cm and diameter 8 cm. Two equal conical hole have been made from its both ends. If the diameter of the holes is 6 cm and height 4 cm, find (i) volume of the cylinder, (ii) volume of one conical hole, (iii) volume of the remaining solid.
A circus tent is cylindrical to a height of 3 m and conical above it. If its base radius is 52.5 m and the slant height of the conical portion is 53 m, find the area of canvas needed to make the tent.
In a corner of a rectangular field with dimensions 35m × 22 m, a well with 14 m inside diameter is dug 8 m deep. The earth dug out is spread evenly over the remaining part of the field. Find the rise in the level of the field.
66 cubic cm of silver is drawn into a wire 1 mm in diameter. Calculate the length of the wire in metres.
Choose the correct answer of the following question:
A solid is hemispherical at the bottom and conical (of same radius) above it. If the surface areas of the two parts are equal, then the ratio of its radius and the slant height of the conical part is
The area of the base of a right circular cone is 154 cm2 and its height is 14 cm. Its curved surface area is
The radius of the base and the height of a solid right circular cylinder are in the ratio 2 : 3 and its volume is 1617 cm3. Find the total surface area of the cylinder.
