Advertisements
Advertisements
प्रश्न
A cylinder of circumference 8 cm and length 21 cm rolls without sliding for `4 1/2` seconds at the rate of 9 complete rounds per second. Find the distance travelled by the cylinder in `4 1/2` seconds.
उत्तर
Circumference of cylinder = 8 cm
Therefore, radius = `c/(2pi) = (8 xx 7)/(2 xx 22) = 14/11 cm`
Length of the cylinder (h) = 21 cm

If distance covered in one revolution is 8 cm, then distance covered in 9 revolution = 9 × 8 = 72
Therefore, distance covered in `4 1/2` seconds
= `72 xx 9/2 cm`
= 324 cm
APPEARS IN
संबंधित प्रश्न
It costs Rs 2200 to paint the inner curved surface of a cylindrical vessel 10 m deep. If the cost of painting is at the rate of Rs 20 per m2, find
(i) Inner curved surface area of the vessel
(ii) Radius of the base
(iii) Capacity of the vessel
`["Assume "pi=22/7]`
The capacity of a closed cylindrical vessel of height 1 m is 15.4 litres. How many square metres of metal sheet would be needed to make it?
`["Assume "pi=22/7]`
A cylindrical boiler, 2 m high, is 3.5 m in diameter. It has a hemispherical lid. Find the volume of its interior, including the part covered by the lid.
An open cylindrical vessel of internal diameter 7 cm and height 8 cm stands on a horizontal table. Inside this is placed a solid metallic right circular cone, the diameter of whose base is `3 1/2` cm and height 8 cm. Find the volume of water required to fill the vessel. If this cone is replaced by another cone, whose height is `1 3/4` cm and the radius of whose base is 2 cm, find the drop in the water level.
Find the total surface area of an open pipe of length 50 cm, external diameter 20 cm and internal diameter 6 cm.
The difference between the outer curved surface area and the inner curved surface area of a hollow cylinder is 352 cm2. If its height is 28 cm and the volume of material in it is 704 cm3; find its external curved surface area.
If the height of a cylinder becomes `1/4` of the original height and the radius is doubled, then which of the following will be true?
Two cylinders of equal volume have heights in the ratio 1 : 9. The ratio of their radii is ______.
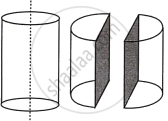
A solid metallic cylinder is cut into two identical halves along its height (as shown in the diagram). The diameter of the cylinder is 7 cm and the height is 10 cm.
Find:
- The total surface area (both the halves).
- The total cost of painting the two halves at the rate of ₹ 30 per cm2 `("Use" π = 22/7)`.
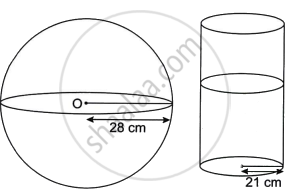
Oil is stored in a spherical vessel occupying `3/4` of its full capacity. Radius of this spherical vessel is 28 cm. This oil is then poured into a cylindrical vessel with a radius of 21 cm. Find the height of the oil in the cylindrical vessel (correct to the nearest cm). Take π = `22/7`