Advertisements
Advertisements
प्रश्न
A cylindrical boiler, 2 m high, is 3.5 m in diameter. It has a hemispherical lid. Find the volume of its interior, including the part covered by the lid.
उत्तर
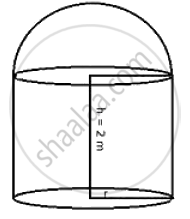
Diameter of cylindrical boiler = 3.5 m
∴ Radius (r) = `3.5/2 = 35/20 = 7/4 m `
Height (h) = 2 m
Radius of hemisphere (R) = `7/4 m `
Total volume of the boiler = `pir^2h + 2/3pir^3`
= `pir^2(h + 2/3r)`
= `22/7 xx 7/7 xx 7/4(2 + 2/3 xx 7/4)`
= `77/8(2 + 7/6)`
= `77/8 xx 19/6`
= `1463/48`
= 30.48 m3
APPEARS IN
संबंधित प्रश्न
A metal container in the form of a cylinder is surmounted by a hemisphere of the same radius. The internal height of the cylinder is 7 m and the internal radius is 3.5 m.
Calculate:
- the total area of the internal surface, excluding the base;
- the internal volume of the container in m3.
A test tube consists of a hemisphere and a cylinder of the same radius. The volume of the water required to fill the whole tube is `5159/6 cm^3` and `4235/6 cm^3` of water is required to fill the tube to a level which is 4 cm below the top of the tube. Find the radius of the tube and the length of its cylindrical part.
How many cubic meters of earth must be dug out to make a well 28 m deep and 2.8 m in diameter? Also, find the cost of plastering its inner surface at Rs. 4.50 per sq.meter.
How much iron is needed to make a rod of length 90 cm and diameter 1.4 cm?
The barrel of a fountain-pen cylindrical in shape is 7 cm long and 5 mm in diameter. A full barrel of ink in the pen will be used for writing 330 words on an average. How many words can be written using a bottle of ink containing one-fifth of a litre?
A small village, having a population of 5000, requires 75 litres of water per head per day. The village has got an overhead tank of measurement 40 m × 25 m × 15 m. For how many days will the water of this tank last?
If the height of a cylinder becomes `1/4` of the original height and the radius is doubled, then which of the following will be true?
The dimensions of a godown are 40 m, 25 m and 10 m. If it is filled with cuboidal boxes each of dimensions 2 m × 1.25 m × 1 m, then the number of boxes will be ______.
The volume of a cylinder which exactly fits in a cube of side a is ______.
Metallic discs of radius 0.75 cm and thickness 0.2 cm are melted to obtain 508.68 cm3 of metal. Find the number of discs melted (use π = 3.14).
