Advertisements
Advertisements
प्रश्न
A circus tent is cylindrical to a height of 8 m surmounted by a conical part. If total height of the tent is 13 m and the diameter of its base is 24 m; calculate :
- total surface area of the tent,
- area of canvas, required to make this tent allowing 10% of the canvas used for folds and stitching.
उत्तर
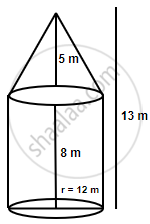
Height of the cylindrical part = H = 8 m
Height of the conical part = h = (13 – 8) m = 5 m
Diameter = 24 m ⟶ radius = r = 12 m
Slant height of the cone = l
`l = sqrt(r^2 + h^2)`
`l = sqrt(12^2 + 5^2)`
`l = sqrt(169) = 13 m`
Slant height of cone = 13 m
i. Total surface are of the tent
= 2πrh + πrl
= πr(2h + l)
= `22/7 xx 12 xx (2 xx 8 + 13)`
= `264/7(16 + 13)`
= `264/7 xx 29`
= `7656/7 m^2`
= 1093.71 m2
ii. Area of canvas used in stitching = Total area
Total area of canvas = `7656/7 + "Total area of canvas"/10`
`=>` Total area of canvas – `"Total area of canvas"/10 = 7656/7`
`=> "Total area of canvas" (1 - 1/10) = 7656/7`
`=> "Total area of canvas" xx 9/10 = 7656/7`
`=>` Total area of canvas = `7656/7 xx 10/9`
`=>` Total area of canvas = `76560/63 = 1215.23 m^2`
APPEARS IN
संबंधित प्रश्न
Water flows, at 9 km per hour, through a cylindrical pipe of cross-sectional area 25 cm2. If this water is collected into a rectangular cistern of imensions 7.5 m by 5 m by 4 m; calculate therise in level in the cistern in 1 hour 15 minutes.

Calculate:
(a) the total surface area,
(b) the total volume of the solid and
(c) the density of the material if its total weight is 1.7 kg..
3080 cm3 of water is required to fill a cylindrical vessel completely and 2310 cm3 of water is required to fill it upto 5 cm below the top. Find :
- radius of the vessel.
- height of the vessel.
- wetted surface area of the vessel when it is half-filled with water.
Water is being pumped out through a circular pipe whose external diameter is 7 cm. If the flow of water is 72 cm per second how many litres of water are being pumped out in one hour.
A right-angled triangle whose sides are 6 cm, 8 cm and 10 cm is revolved about the sides containing the right angle in two ways. Find the difference in volumes of the two solids so formed.
If the radius of the base of a right circular cylinder is halved keeping the same height, then the ratio of the volume of the cylinder thus obtained to the volume of original cylinder is
The volume of the largest right circular cone that can be fitted in a cube whose edge is 2r equals to the volume of a hemisphere of radius r.
A school provides milk to the students daily in a cylindrical glasses of diameter 7 cm. If the glass is filled with milk upto an height of 12 cm, find how many litres of milk is needed to serve 1600 students.
The volume of a cylinder becomes ______ the original volume if its radius becomes half of the original radius.
The thickness of a hollow metallic cylinder is 2 cm. It is 70 cm long with outer radius of 14 cm. Find the volume of the metal used in making the cylinder, assuming that it is open at both the ends. Also find its weight if the metal weighs 8 g per cm3.
A rectangular sheet of paper is rolled in two different ways to form two different cylinders. Find the volume of cylinders in each case if the sheet measures 44 cm × 33 cm.
