Advertisements
Advertisements
प्रश्न
A horse is placed for grazing inside a rectangular field 40m by 36m and is tethered to one corner by a rope 14m long. Over how much area can it graze.
उत्तर

The fig shows rectangular field ABCD at corner A, a horse is tied with rope length = 14m.
The area it can graze is represented A as shaded region= area of quadrant with (radius = length) of string
Area = `1/4`× (𝑎𝑟𝑒𝑎 𝑜𝑓 𝑐𝑖𝑟𝑐𝑙𝑒) = 𝜋𝑟2
`= 1/4 ×22/7 × 14 × 14`
= (22 × 7)
= 154 m2.
Area it can graze = 154m2.
APPEARS IN
संबंधित प्रश्न
The radius of a circle is 7 cm. find the circumference of the circle.
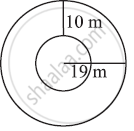
Find the circumference of the inner and the outer circles, shown in the adjoining figure? (Take π = 3.14)
Choose the correct alternative answer for the following question.
The curved surface area of a cylinder is 440 cm 2 and its radius is 5 cm. Find its height.
A horse is placed for grazing inside a rectangular field 70 m by 52 m. It is tethered to one corner by a rope 21 m long. On how much area can it graze? How much area is left ungrazed?
The diameter of a wheel is 84 cm. How many revolutions will it make to cover 792 m?
Find the curved surface area , the total surface area and the volume of a cone if its :
Height = 15 cm , radius = 8 cm
The sum of the circumference and diameter of a circle is 116 cm. Find its radius.
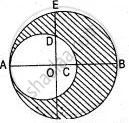
In the adjoining figure, the crescent is formed by two circles which touch at the point A. O is the centre of bigger circle. If CB = 9 cm and DE = 5 cm, find the area of the shaded portion.
Find the area and perimeter of the circles with following: Radius = 10.5cm
The circumference of a circle is equal to the perimeter of a square. The area of the square is 484 sq.m. Find the area of the circle.
