Advertisements
Advertisements
प्रश्न
A horse is placed for grazing inside a rectangular field 70 m by 52 m. It is tethered to one corner by a rope 21 m long. On how much area can it graze? How much area is left ungrazed?
उत्तर
Radius of the quadrant of the circle = 21 m
The shaded portion shows the part of the field the horse can graze.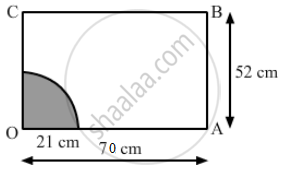
Area of the grazed field = Area of the quadrant OPQ
`=1/4` of the circle having radius OP
`=1/4pi"r"^2`
`=1/4xxpi"r"^2`
`= 1/4 xx 22/7xx21xx21`
= 346.5 m2
Total area of the field = 70 × 52 = 3640 m2
Area left ungrazed = Area of the field -- Area of the grazed field
= 3640 - 346.5 = 3293.5 m2
APPEARS IN
संबंधित प्रश्न
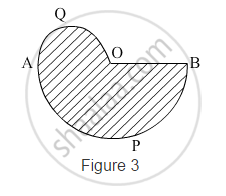
In Fig. 3, APB and AQO are semicircles, and AO = OB. If the perimeter of the figure is 40 cm, find the area of the shaded region [Use `pi=22/7`]
The base of a right – angled triangle measures 48 cm and its hypotenuse measures 50 cm. Find the area of the triangle.
The radius of the circle is given below; find the area.
28 cm
Find the area and perimeter of the circles with following: Radius = 10.5cm
Find the area of a circular field that has a circumference of 396m.
The circumference of a circle exceeds its diameter by 450 cm. Find the area of the circle.
Find the perimeter of the given shape (Take π = `22/7`).
Diameters of different circles are given below. Find their circumference (Take π = `22/7`)
d = 28 mm
Areas of two circles are equal. Is it necessary that their circumferences are equal? Why?
A dinner plate is in the form of circle. A circular region encloses a beautiful design as shown in the given figure. The inner circumference is 352 mm and outer is 396 mm. Find the width of circular design.

