Advertisements
Advertisements
प्रश्न
A magnetic field in a certain region is given by `B = B_o cos (ωt)hatk` and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field (Figure) . Find the magnitude and the direction of the current at (a, 0, 0) at t = π/2ω, t = π/ω and t = 3π/2ω.
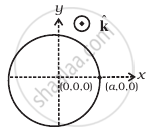
उत्तर
- First law: Whenever the number of magnetic lines of force (magnetic flux) passing through a circuit changes, an emf is produced in the circuit called induced emf. The induced emf persists only as long as there is a change or cutting of flux.
- Second law: The induced emf is given by the rate of charge of magnetic flux linked with the circuit, i.e, `e = - (dphi)/(dt)`. For N turns `e = - (N dphi)/(dt)`; NEgative sign indicates that induced emf (e) opposes the change of flux.
First we need to find out the flux passing through the ring at any instant and that is given by
`phi_m = vecB * vecA = BA cos theta`
And as we know both `vecA` (area vector) and `vecB` (magnetic field vector) are directed along z-axis. So, angle between them is 0.
So, cos θ = 1 .....(∵ θ = 0)
⇒ `phi_m = BA`
Area of coil of radius a = πa2
ε = `B_0(pia^2) cos ωt`
By Faraday's law of electromagnetic induction,
Magnitude of induced emf is given by ε = `B_0(pia^2) ω sin ωt`
This causes flow of indeed current, which is given by `I = (B_0(pia^2) ω sin ωt)/R`
Now, the value of current at different instants,
(i) `t = pi/(2ω)`
`I = (B_0(pia^2)ω)/R` along `hatj`
Because sin ωt = `sin(ω pi/(2ω)) = sin pi/2` = 1
(ii) `t = pi/ω, I = (B(pia^2)ω)/R` = 0
Because, sin ωt = `sin(ω pi/ω) = sin pi` = 0
(iii) `t = 3/2 pi/ω`
`I = (B(pia^2)ω)/R` along `-hatj`
sin ωt = `sin(ω * (3pi)/(2ω)) = sin (3pi)/2` = – 1
APPEARS IN
संबंधित प्रश्न
A .0.5m long solenoid of 10 turns/cm has area of cross-section 1cm2 . Calculate the voltage induced across its ends if the current in the solenoid is changed from 1A to 2A in 0.1s.
A 0⋅5 m long solenoid of 10 turns/cm has the area of cross-section 1 cm2. Calculate the voltage induced across its ends if the current in the solenoid is changed from 1 A to A in 0⋅1 s.
The two rails of a railway track, insulated from each other and the ground, are connected to millivoltmeter. What is the reading of the millivoltmeter when a train passes at a speed of 180 km/hr along the track, given that the vertical component of earth’s magnetic field is 0.2 × 10–4 wb/m2 and rails are separated by 1 metre ______.
According to Faraday’s law of electromagnetic induction ______.
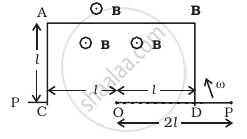
ODBAC is a fixed rectangular conductor of negilible resistance (CO is not connnected) and OP is a conductor which rotates clockwise with an angular velocity ω (Figure). The entire system is in a uniform magnetic field B whose direction is along the normal to the surface of the rectangular conductor ABDC. The conductor OP is in electric contact with ABDC. The rotating conductor has a resistance of λ per unit length. Find the current in the rotating conductor, as it rotates by 180°.
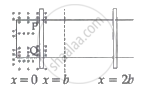
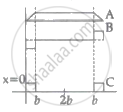
The arm PQ of a rectangular conductor is moving from x = 0 to x = 2b outwards and then inwards from x = 2b to x = 0 as shown in the figure. A uniform magnetic field perpendicular to the plane is acting from x = 0 to x = b. Identify the graph showing the variation of different quantities with distance.
The self induced emf of a coil is 25 volts. When the current in it is changed at uniform rate from 10 A to 25 A in 1 s, the change in the energy of the inductance is ______.
A square-shaped coil of side 10 cm, having 100 turns is placed perpendicular to a magnetic field which is increasing at 1 T/s. The induced emf in the coil is ______.
Two coils C1 and C2 are placed close to each other. The magnetic flux Φ2 linked with coil C2 varies with the current I1 flowing in coil C1 as shown in the figure. Find

- The mutual inductance of the arrangement, and
- The rate of change of current `((dI_1)/(dt))` will induce an emf of 100V in coil C2.
How much charge in terms of Faraday is required for the reduction of 1 mol of Cu2+ to Cu?
