Advertisements
Advertisements
Question
A magnetic field in a certain region is given by `B = B_o cos (ωt)hatk` and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field (Figure) . Find the magnitude and the direction of the current at (a, 0, 0) at t = π/2ω, t = π/ω and t = 3π/2ω.
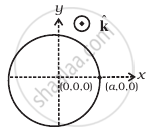
Solution
- First law: Whenever the number of magnetic lines of force (magnetic flux) passing through a circuit changes, an emf is produced in the circuit called induced emf. The induced emf persists only as long as there is a change or cutting of flux.
- Second law: The induced emf is given by the rate of charge of magnetic flux linked with the circuit, i.e, `e = - (dphi)/(dt)`. For N turns `e = - (N dphi)/(dt)`; NEgative sign indicates that induced emf (e) opposes the change of flux.
First we need to find out the flux passing through the ring at any instant and that is given by
`phi_m = vecB * vecA = BA cos theta`
And as we know both `vecA` (area vector) and `vecB` (magnetic field vector) are directed along z-axis. So, angle between them is 0.
So, cos θ = 1 .....(∵ θ = 0)
⇒ `phi_m = BA`
Area of coil of radius a = πa2
ε = `B_0(pia^2) cos ωt`
By Faraday's law of electromagnetic induction,
Magnitude of induced emf is given by ε = `B_0(pia^2) ω sin ωt`
This causes flow of indeed current, which is given by `I = (B_0(pia^2) ω sin ωt)/R`
Now, the value of current at different instants,
(i) `t = pi/(2ω)`
`I = (B_0(pia^2)ω)/R` along `hatj`
Because sin ωt = `sin(ω pi/(2ω)) = sin pi/2` = 1
(ii) `t = pi/ω, I = (B(pia^2)ω)/R` = 0
Because, sin ωt = `sin(ω pi/ω) = sin pi` = 0
(iii) `t = 3/2 pi/ω`
`I = (B(pia^2)ω)/R` along `-hatj`
sin ωt = `sin(ω * (3pi)/(2ω)) = sin (3pi)/2` = – 1
APPEARS IN
RELATED QUESTIONS
A jet plane is travelling towards west at a speed of 1800 km/h. What is the voltage difference developed between the ends of the wing having a span of 25 m, if the Earth’s magnetic field at the location has a magnitude of 5 × 10−4 T and the dip angle is 30°.
State Faraday's first law of electrolysis.
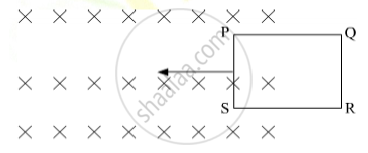
The closed loop (PQRS) of wire is moved into a uniform magnetic field at right angles to the plane of the paper as shown in the figure, Predict the direction of the induced current in the loop.
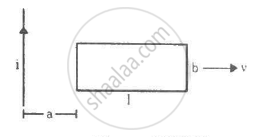
A rectangular metallic loop of length l and width b is placed coplanarly with a long wire carrying a current i (figure). The loop is moved perpendicular to the wire with a speed vin the plane containing the wire and the loop. Calculate the emf induced in the loop when the rear end of the loop is at a distance a from the wire. solve by using Faraday's law for the flux through the loop and also by replacing different segments with equivalent batteries.
E°cell for the given redox reaction is 2.71V
\[\ce{Mg_{(s)} +Cu^{2+}_{(0.01 M)}->Mg^{2+}_{(0.001M)}+Cu_{(s)}}\]
Calculate Ecell for the reaction. Write the direction of flow of current when an external opposite potential applied is
(i) less than 2.71 V and
(ii) greater than 2.71 V
Faraday’s laws are consequence of the conservation of ______.
The average e.m.f induced in a coil in which current change from 0.2 ampere to 0.4 ampere in· 0.1 sec is 1 volt, the self-inductance of the coil is
According to Faraday's law of electromagnetic induction, ______
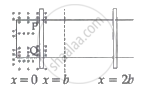
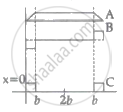
The arm PQ of a rectangular conductor is moving from x = 0 to x = 2b outwards and then inwards from x = 2b to x = 0 as shown in the figure. A uniform magnetic field perpendicular to the plane is acting from x = 0 to x = b. Identify the graph showing the variation of different quantities with distance.
Two coils C1 and C2 are placed close to each other. The magnetic flux Φ2 linked with coil C2 varies with the current I1 flowing in coil C1 as shown in the figure. Find

- The mutual inductance of the arrangement, and
- The rate of change of current `((dI_1)/(dt))` will induce an emf of 100V in coil C2.
