Question

A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively is melted and recast into the form a cone of base diameter 8 cm. The height of the cone is ______.

पर्याय

• 12 cm

• 14 cm

• 15 cm

• 18 cm

Answer 1

A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively is melted and recast into the form a cone of base diameter 8cm. The height of the cone is 14 cm.

Explanation: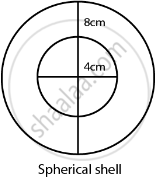

Volume of spherical shell = Volume of cone recast by melting

For spherical shell,

Internal diameter, d₁ = 4 cm

Internal radius, r₁ = 2 cm  ...[As radius = `1/2` diameter]

External diameter, d₂ = 8 cm

External radius, r₂ = 4 cm

Now,

As volume of spherical shell= `4/3 π("r"_2^3 - "r"_1^3)`

Where r₁ and r₂ are internal and external radii respectively.

Volume of given shell = `4/3 π(4^3 - 2^3)`

= `4/3 π(56)`

= `(224/3)π`

We know that,

Volume of cone = `(224π)/3 "cm"^3`

For cone,

Base diameter = 8 cm

Base radius, r = 4 cm

Let Height of cone = ‘h’.

We know,

Volume of cone = `(1/3)π "r"^2"h"`,

Where r = Base radius and h = Height of cone

Volume of given cone = `(1/3)π4^2"h"`

⇒ `(224π)/3 = (16π"h")/3`

⇒ 16h = 224

h = 14 cm

So, Height of cone is 14 cm.