Advertisements
Advertisements
प्रश्न
A population of 200 fruit flies is in Hardy Weinberg equilibrium. The frequency of the allele (a) 0.4. Calculate the following:
The number of carrier fruit flies.
उत्तर
In Hardy Weinberg, p2 + 2pq + q2 = 1, where 'p2' is the frequency of the homozygous dominant genotype (AA), '2pq' is the frequency of the heterozygous genotype (Aa), and 'q2' is the frequency of the homozygous recessive genotype (aa).
Given:
q = 0.4
We know p + q = 1
p = 1 − q
= 1 − 0.4
= 0.6
Number of carrier fruit flies is
As, 2pq (Aa)
= 2 × 0.6 × 0.4
= 0.48
= 0.48 × 200
= 96
APPEARS IN
संबंधित प्रश्न
What is the Founder's effect?
How does the Hardy-Wienberg equation explain genetic equilibrium?
A population will not exist in Hardly Weiberg equilibrium if ____________.
In the Hardy-Weinberg equilibrium equation, the homozygous mutant is represented as ______.
Disturbance of Hardy - Weinberg equilibrium results in
For the MN-blood group system, the frequencies of M and N alleles are 0.7 and 0.3, respectively. The expected frequency of MN-blood group bearing organisms is likely to be ______.
List any two factors that can disturb the genetic equilibrium.
The graphs below show three types of natural selection. The shaded areas marked with arrows show the individuals in the population who are not selected. The dotted vertical lines show the statistical means.
 |
 |
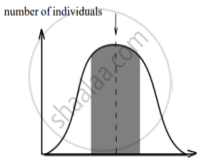 |
| character Graph A |
character Graph B |
character Graph C |
- What names are given to the types of selection shown in graphs A, B and C?
- After the selection has operated for several generations in the above populations indicated as, Graph A, B and C, graphically illustrate the probable results.
Explain Hardy-Weinberg's principle
A population of 200 fruit flies is in Hardy Weinberg equilibrium. The frequency of the allele (a) 0.4. Calculate the following:
Frequency of the allele (A).
