Advertisements
Advertisements
प्रश्न
A rectangular frame is to be suspended symmetrically by two strings of equal length on two supports (Figure). It can be done in one of the following three ways;
| (a) | 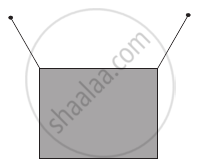 |
| (b) |  |
| (c) | 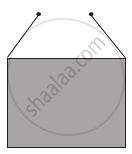 |
The tension in the strings will be ______.
पर्याय
the same in all cases.
least in (a).
least in (b).
least in (c).
उत्तर
The tension in the strings will be least in (b).
Explanation:
Consider the FBD diagram of the rectangular frame
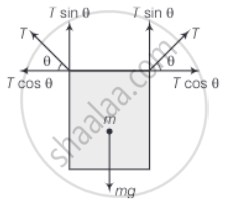
Balancing vertical forces 2T sin θ – mg = 0 ......[T is tension in the string]
⇒ 2T sin θ = mg ......(i)
Total horizontal force = T cos θ – T cos θ = 0
Now from equation (i), T = `(mg)/(2 sin θ)`
As mg is constant
⇒ `T ∝ 1/sin θ`
⇒ `T_(max) = (mg)/(2 sin θ_(min))`
`sin θ_(min)` = 0
⇒ `θ_(min)` = 0
No option matches with θ = 0°
`T_(min) = (mg)/(2 sin θ_(max))` ......(since, sin θmax = 1)
sin θmax = 1
⇒ θ = 90°
Matches with option (b)
Hence, tension is least for case (b).
APPEARS IN
संबंधित प्रश्न
Two strips of metal are riveted together at their ends by four rivets, each of diameter 6.0 mm. What is the maximum tension that can be exerted by the riveted strip if the shearing stress on the rivet is not to exceed 6.9 × 107 Pa? Assume that each rivet is to carry one-quarter of the load.
A wire can sustain the weight of 20 kg before breaking. If the wire is cut into two equal parts, each part can sustain a weight of
A rod has a radius of 100 mm and a length of 10 cm. A 100 N force compress along its length. Calculate the longitudinal stress developed in the rod.
Modulus of rigidity of ideal liquids is ______.
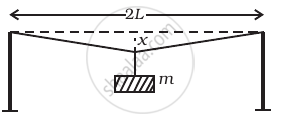
A mild steel wire of length 2L and cross-sectional area A is stretched, well within elastic limit, horizontally between two pillars (Figure). A mass m is suspended from the mid point of the wire. Strain in the wire is ______.
A rod of length l and negligible mass is suspended at its two ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths (Figure). The cross-sectional areas of wires A and B are 1.0 mm2 and 2.0 mm2, respectively.
(YAl = 70 × 109 Nm−2 and Ysteel = 200 × 109 Nm–2)
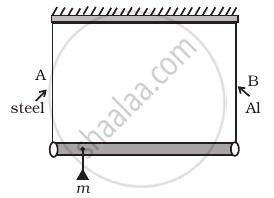
- Mass m should be suspended close to wire A to have equal stresses in both the wires.
- Mass m should be suspended close to B to have equal stresses in both the wires.
- Mass m should be suspended at the middle of the wires to have equal stresses in both the wires.
- Mass m should be suspended close to wire A to have equal strain in both wires.
Consider a long steel bar under a tensile stress due to forces F acting at the edges along the length of the bar (Figure). Consider a plane making an angle θ with the length. What are the tensile and shearing stresses on this plane
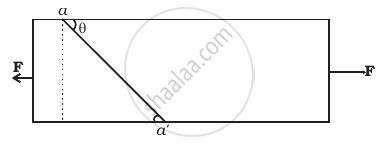
- For what angle is the tensile stress a maximum?
- For what angle is the shearing stress a maximum?
The value of tension in a long thin metal wire has been changed from T1 to T2. The lengths of the metal wire at two different values of tension T1 and T2 are l1 and l2 respectively. The actual length of the metal wire is ______.
A steel wire having a radius of 2.0 mm, carrying a load of 4 kg, is hanging from a ceiling. Given that g = 3.1πms-2, what will be the tensile stress that would be developed in the wire?
A body of mass m = 10 kg is attached to one end of a wire of length 0.3 m. The maximum angular speed (in rad s-1) with which it can be rotated about its other end in the space station is (Breaking stress of wire = 4.8 × 107 Nm-2 and the area of cross-section of the wire = 10-2 cm2) is ______.
