Advertisements
Advertisements
प्रश्न
A star like the sun has several bodies moving around it at different distances. Consider that all of them are moving in circular orbits. Let r be the distance of the body from the centre of the star and let its linear velocity be v, angular velocity ω, kinetic energy K, gravitational potential energy U, total energy E and angular momentum l. As the radius r of the orbit increases, determine which of the above quantities increase and which ones decrease.
उत्तर
The situation is shown in the diagram, where a body of mass m is revolving around a star of mass M.
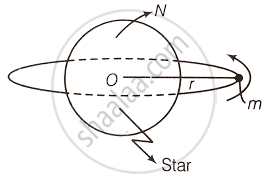
Linear velocity of the body `v = sqrt((GM)/r)`
⇒ `v ∝ 1/sqrt(r)`
Therefore, when r increases, v decreases.
Angular velocity of the body `ω = (2π)/T`
According to Kepler's law of the period,
`T^2 ∝ r^3` ⇒ `T = kr^(3/2)`
Where k is a constant
∴ `ω = (2π)/(kr^(3/2))` ⇒ `ω ∝ 1/r^(3/2)` .....`(∵ ω = (2π)/T)`
Therefore, when r increases, ω decreases.
The kinetic energy of the body,
K = `1/2 mv^2`
= `1/2 m xx (GM)/r`
= `(GMm)/(2r)`
∴ `K ∝ 1/r`
Therefore, when r increases, KE decreases.
The gravitational potential energy of the body,
`U = - (GMm)/r` ⇒ `U ∝ 1/r`
Therefore, when r increases, PE becomes less negative i.e., increases.
Total energy of the body,
`E = KE + PE`
= `(GMm)/(2r) + (- (GMm)/r)`
= `- (GMm)/(2r)`
Therefore, when r increases, total energy becomes less negative, i.e., increases.
Angular momentum of the body,
`L = mvr`
= `mr sqrt((GM)/r)`
= `msqrt(GMr)`
∴ `L ∝ sqrt(r)`
Therefore, when r increases, angular momentum L increases.
APPEARS IN
संबंधित प्रश्न
State Kepler's laws of planetary motion.
Both earth and moon are subject to the gravitational force of the sun. As observed from the sun, the orbit of the moon ______.
In our solar system, the inter-planetary region has chunks of matter (much smaller in size compared to planets) called asteroids. They ______.
If the sun and the planets carried huge amounts of opposite charges ______.
- all three of Kepler’s laws would still be valid.
- only the third law will be valid.
- the second law will not change.
- the first law will still be valid.
If the sun and the planets carried huge amounts of opposite charges ______.
- all three of Kepler’s laws would still be valid.
- only the third law will be valid.
- the second law will not change.
- the first law will still be valid.
Supposing Newton’s law of gravitation for gravitation forces F1 and F2 between two masses m1 and m2 at positions r1 and r2 read F1 = – F2 = `- r_12/r_12^3 GM_0^2 ((m_1m_2)/M_0^2)^n` where M0 is a constant of dimension of mass r12 = r1 – r2 and n is a number. in such a case.
- the acceleration due to gravity on earth will be different for different objects.
- none of the three laws of Kepler will be valid.
- only the third law will become invalid.
- for n negative, an object lighter than water will sink in water.
Give one example each of central force and non-central force.
A satellite is in an elliptic orbit around the earth with aphelion of 6R and perihelion of 2 R where R= 6400 km is the radius of the earth. Find eccentricity of the orbit. Find the velocity of the satellite at apogee and perigee. What should be done if this satellite has to be transferred to a circular orbit of radius 6R ?
[G = 6.67 × 10–11 SI units and M = 6 × 1024 kg]
A planet revolving in an elliptical orbit has:
- a constant velocity of revolution.
- has the least velocity when it is nearest to the sun.
- its areal velocity is directly proportional to its velocity.
- areal velocity is inversely proportional to its velocity.
- to follow a trajectory such that the areal velocity is constant.
Choose the correct answer from the options given below:
lf the angular momentum of a planet of mass m, moving around the Sun in a circular orbit is L, about the center of the Sun, and its areal velocity is ______.
