Advertisements
Advertisements
प्रश्न
A thin straight infinitely long conducting wire having charge density λ is enclosed by a cylindrical surface of radius r and length l, its axis coinciding with the length of the wire. Find the expression for the electric flux through the surface of the cylinder.
उत्तर
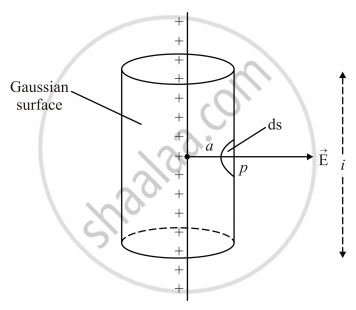
The thin infinitely long straight line has a linear charge density λ.
Since the electric field for this kind of configuration will be radial and perpendicular to the wire, there will be no flux through the flat surfaces of the cylinder. Also, the electric field (E) will be constant at every point on the curved surface of the cylinder (as all points on it are equidistant from the wire) and perpendicular to it.
We shall us Gauss's law to find the electric flux through the cylinder. The charge enclosed by the cylinder is λ × l, as l is the length of the cylinder and it is also the length of the charged wire within the cylinder.
We know,
`\text { Electric flux} = (\text { Charge enclosed})/epsi_0 = (lambdal)/epsi_0`
APPEARS IN
संबंधित प्रश्न
What is the electric flux through a cube of side 1 cm which encloses an electric dipole?
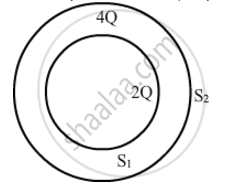
Consider two hollow concentric spheres, S1 and S2, enclosing charges 2Q and 4Q respectively as shown in the figure. (i) Find out the ratio of the electric flux through them. (ii) How will the electric flux through the sphere S1 change if a medium of dielectric constant 'εr' is introduced in the space inside S1 in place of air ? Deduce the necessary expression
Careful measurement of the electric field at the surface of a black box indicates that the net outward flux through the surface of the box is 8.0 × 103 N m2/C.
- What is the net charge inside the box?
- If the net outward flux through the surface of the box were zero, could you conclude that there were no charges inside the box? Why or Why not?
Two charges of magnitudes −2Q and +Q are located at points (a, 0) and (4a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘3a’ with its centre at the origin?
Two charges of magnitudes +4Q and − Q are located at points (a, 0) and (− 3a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘2a’ with its centre at the origin?
A small plane area is rotated in an electric field. In which orientation of the area, is the flux of the electric field through the area maximum? In which orientation is it zero?
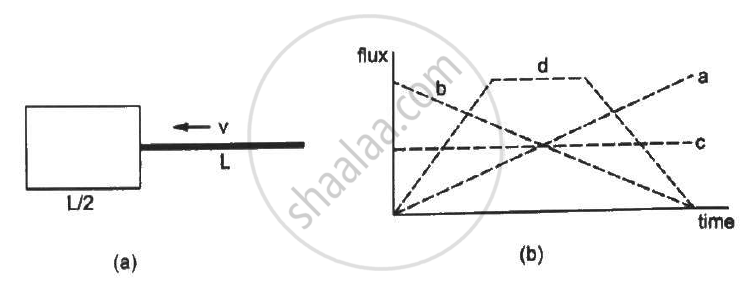
Following Figure (a) shows an imaginary cube of edge L/2. A uniformly charged rod of length (L) moves towards the left at a small but constant speed `nu.` At t = 0, the left end just touches the centre of the face of the cube opposite it. Which of the graphs shown in the figure (b) represents the flux of the electric field through the cube as the rod goes through it?
A charge q is placed at the centre of the open end of a cylindrical vessel (see the figure). The flux of the electric field through the surface of the vessel is ____________ .

The S.I. unit of electric flux is ______
A circular disc of radius 'r' is placed along the plane of paper. A uniform electric field `vec"E"` is also present in the plane of paper. What amount of electric flux is associated with it?