Advertisements
Advertisements
प्रश्न
A well of diameter 3 m is dug 14 m deep. The earth taken out of it has been spread evenly all around it in the shape of a circular ring of width 4 m to form an embankment. Find the height of the embankment.
A well of diameter 3 m is dug 14 m deep. The earth taken out of it has been spread evenly all around it to a width of 4 m to form an embankment. Find the height of the embankment.
उत्तर
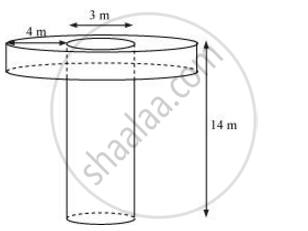
The shape of the well will be cylindrical.
Depth (h1) of well = 14 m
Radius (r1) of the circular end of well = 3/2 m
Width of embankment = 4 m
From the figure, it can be observed that our embankment will be in a cylindrical shape having outer radius (r2) as 4 + 3/2 =11/2 m and inner radius (r1) as 3/2 m
Let the height of embankment be h2.
Volume of soil dug from well = Volume of earth used to form embankment
`pixxr_1^2xxh_1 = pixx(r_2^2-r_1^2)xxh_2`
`pixx(3/2)^2xx 14 =pixx[(11/2)^2-(3/2)^2]xxh`
`9/4xx14 = 112/4 xx h`
h = 9/8 = 1.125 m
Therefore, the height of the embankment will be 1.125 m.
संबंधित प्रश्न
A tent is in the form of a cylinder of diameter 20 m and height 2.5 m, surmounted by a cone of equal base and height 7.5 m. Find the capacity of the tent and the cost of the canvas at Rs 100 per square metre.
A rectangular sheet of paper 40 cm × 22 cm, is rolled to form a hollow cylinder of height 40 cm. The radius of the cylinder (in cm) is ______.
Two cylindrical vessels are filled with oil. Their radii are 15 cm, 12 cm and heights 20 cm, 16 cm respectively. Find the radius of a cylindrical vessel 21 cm in height, which will just contain the oil of the two given vessels.
The height of a cone is 30 cm. A small cone is cut off at the top by a plane parallel to the base. If its volume be \[\frac{1}{27}\] of the volume of the given cone, then the height above the base at which the section has been made, is
The diameter of a cylinder is 28 cm and its height is 20 cm. The total surface area of the cylinder is
The curved surface are of a cylinder is 1760 cm2 and its base radius is 14 cm. The height of the cylinder is
______ surface area of room = area of 4 walls.
The length, breadth and height of a cuboidal reservoir is 7 m, 6 m and 15 m respectively. 8400 L of water is pumped out from the reservoir. Find the fall in the water level in the reservoir.
If the length of the diagonal of a cube is `5sqrt(3)` cm, find the total surface area.
Length of the diagonal of the cube = `square`
So, `square` = `5sqrt(3)`
⇒ Side = `square`
Total surface area of cube = `square`
= `square` × `square` × `square`
= `square` cm2
Hence, the total surface area is `square`.
The surface area of a sphere is 616 sq cm. Find its radius tan β = `3/4`
