Advertisements
Advertisements
प्रश्न
An aircraft executes a horizontal loop at a speed of 720 km/h with its wings banked at 15°. What is the radius of the loop?
उत्तर १
Here v = 720 km/h = `720xx 5/18` m/s = 200 m/s and angle of banking `theta = 15^@`
From the relation
`tan theta = v^2/"rg"` we have
`r = v^2/(g tan theta) = (200xx200) /(10 xx tan 15^@) = (200xx200)/(10xx0.2679)`
=> r = 14931 m = 14.9 km
उत्तर २
Speed of the aircraft, v = 720 km/h = `720xx5/18 = 200` m/s
Acceleration due to gravity, g = 10 m/s2
Angle of banking, θ = 15°
For radius r, of the loop, we have the relation:
`tan theta = v^2/"rg"`
`r = v^2/(g tan theta)`
`= (200xx200)/(10xx tan 15) = 4000/0.268`
= 14925.37 m
= 14.92 km
APPEARS IN
संबंधित प्रश्न
A stone of mass m tied to the end of a string revolves in a vertical circle of radius R. The net forces at the lowest and highest points of the circle directed vertically downwards are: [Choose the correct alternative]
| Lowest Point | Highest Point | |
| a) | mg – T1 | mg + T2 |
| b) | mg + T1 | mg – T2 |
| c) | `mg + T1 –(m_v_1^2)/R` | mg – T2 + (`mv_1^2`)/R |
| d) | `mg – T1 – (mv)/R` | mg + T2 + (mv_1^2)/R |
T1 and v1 denote the tension and speed at the lowest point. T2 and v2 denote corresponding values at the highest point.
In the following figure shows a uniform rod of length 30 cm and mass 3.0 kg. The strings shown in the figure are pulled by constant forces of 20 N and 32 N. Find the force exerted by the 20 cm part of the rod on the 10 cm part. All the surfaces are smooth and the strings and the pulleys are light.

Find the acceleration of the blocks A and B in the three situations shown in the following figure.

A monkey of mass 15 kg is climbing a rope fixed to a ceiling. If it wishes to go up with an acceleration of 1 m/s2, how much force should it apply on the rope? If the rope is 5 m long and the monkey starts from rest, how much time will it take to reach the ceiling?
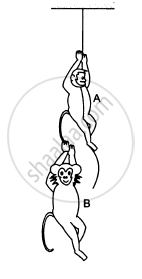
The monkey B, shown in the following figure, is holding on to the tail of monkey A that is climbing up a rope. The masses of monkeys A and B are 5 kg and 2 kg, respectively. If A can tolerate a tension of 30 N in its tail, what force should it apply on the rope in order to carry monkey B with it? Take g = 10 m/s2.
A force of 10 N acts on a body of mass 2 kg for 3 s, initially at rest. Calculate : Change in momentum of the body.
What do you understand by the term momentum?
Multiple Choice Question. Select the correct option.
The impulse of a body is equal to:
ame the law of motion which gives the definition of force.
A metre scale is moving with uniform velocity. This implies ______.
