Advertisements
Advertisements
प्रश्न
An oil funnel made of tin sheet consists of a 10 cm long cylindrical portion attached to a frustum of a cone. If the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, then find the area of the tin sheet required to make the funnel.

उत्तर
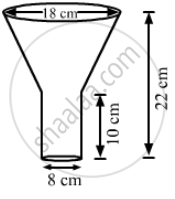
We have,
Height of the cylindrical portion, h = 10 cm,
height of the frustum of cone portion, H = 22 - 10 = 12 cm,
Radius of the cylindrical portion = Radius of smaller end of frustum portion,
Radius of larger end of frustum portion, R = 18/2 = 9 cm
Also, the slant height of the frustum, `l = sqrt(("R - r")^2 + "H"^2)`
`=sqrt((9 - 4)^2+12^2`
`=sqrt(5^2+12^2)`
`=sqrt(25+144`
`=sqrt(169)`
`=13 "cm"`
Now,
The area of the tin sheet required = CSA of frustum of cone + CSAA of cylinder
= π (R + r) l + 2πrh
`= 22/7xx(9+4)x13+2xx22/7xx4xx10`
`=22/7xx13xx13+22/7xx80`
`= 22/7xx(169+80)`
`=22/7xx249`
≈ 782.57 cm2
So, the area of the tin sheet required to make the funnel is 782.57 cm2.
APPEARS IN
संबंधित प्रश्न
A container open at the top, is in the form of a frustum of a cone of height 24 cm with radii of its lower and upper circular ends, as 8 cm and 20 cm respectively. Find the cost of milk which can completely fill the container. at the rate of 21 per litre. [use π=22/7]
The height of a cone is 30 cm. From its topside a small cone is cut by a plane parallel to its base. If volume of smaller cone is `1/27` of the given cone, then at what height it is cut from its base?
A bucket is in the form of a frustum of a cone of height 30 cm with radii of its lower and upper ends as 10 cm and 20 cm respectively. Find the capacity and surface area of the bucket. Also, find the cost of milk which can completely fill the container , at thr rate of ₹25 per litre. (Use \[\pi = 3 . 14) .\]
A cone of radius 4 cm is divided into two parts by drawing a plane through the mid point of its axis and parallel to its base . Compare the volumes of two parts.
A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter `"4"2/3` cm and height 3 cm. Find the number of cones so formed.
The circular ends of a bucket are of radii 35 cm and 14 cm and the height of the bucket is 40 cm. Its volume is
By melting a solid sphere of radius 5 cm a solid right circular cone of the same circular base radius is made. The height of cone is ______.
The shape of a glass (tumbler) (see figure) is usually in the form of ______.
A cone is cut through a plane parallel to its base and then the cone that is formed on one side of that plane is removed. The new part that is left over on the other side of the plane is called ______.
An open metallic bucket is in the shape of a frustum of a cone, mounted on a hollow cylindrical base made of the same metallic sheet. The surface area of the metallic sheet used is equal to curved surface area of frustum of a cone + area of circular base + curved surface area of cylinder.
