Advertisements
Advertisements
प्रश्न
Check if the following relation is function:
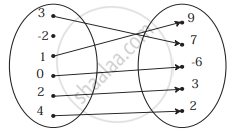
उत्तर
No.
Reason:
Not every element of set A has been assigned an image from set B.
APPEARS IN
संबंधित प्रश्न
A function f : R → R is defined by f(x) = x2. Determine (a) range of f, (b) {x : f(x) = 4}, (c) [y: f(y) = −1].
f, g, h are three function defined from R to R as follow:
(ii) g(x) = sin x
Find the range of function.
If \[f\left( x \right) = x^3 - \frac{1}{x^3}\] , show that
If f, g, h are real functions given by f(x) = x2, g(x) = tan x and h(x) = loge x, then write the value of (hogof)\[\left( \sqrt{\frac{\pi}{4}} \right)\] .
If \[f\left( x \right) = 64 x^3 + \frac{1}{x^3}\] and α, β are the roots of \[4x + \frac{1}{x} = 3\] . Then,
Check if the following relation is function:
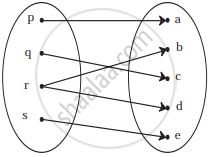
A function f is defined as follows: f(x) = 4x + 5, for −4 ≤ x < 0. Find the values of f(−1), f(−2), f(0), if they exist.
Check if the relation given by the equation represents y as function of x:
x + y2 = 9
Find x, if f(x) = g(x) where f(x) = x4 + 2x2, g(x) = 11x2
Show that if f : A → B and g : B → C are onto, then g ° f is also onto
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
A graph representing the function f(x) is given in it is clear that f(9) = 2

What is the image of 6 under f?
A function f is defined by f(x) = 2x – 3 find x such that f(x) = x
If f(x) = `(x - 1)/(x + 1), x ≠ - 1` Show that f(f(x)) = `- 1/x`, Provided x ≠ 0
Find the domain for which the functions f(x) = 2x2 – 1 and g(x) = 1 – 3x are equal.
Redefine the function which is given by f(x) = `|x - 1| + |1 + x|, -2 ≤ x ≤ 2`
Find the domain of the following function given by:
f(x) = `(3x)/(2x - 8)`
Domain of `sqrt(a^2 - x^2) (a > 0)` is ______.
The domain of the function f defined by f(x) = `sqrt(4 - x) + 1/sqrt(x^2 - 1)` is equal to ______.
Let f(θ) = sin θ (sin θ + sin 3θ) then ______.
