Advertisements
Advertisements
प्रश्न
Choose the correct alternative :
The corner points of the feasible region given by the inequations x + y ≤ 4, 2x + y ≤ 7, x ≥ 0, y ≥ 0, are
पर्याय
(0, 0), (4, 0), (3, 1), (0, 4).
(0, 0), `(7/2, 0)`, (3, 1), (0, 4).
(0, 0), `(7/2, 0), (3, 1)`, (5, 7).
(6, 0), (4, 0), (3, 1), (0, 7).
उत्तर
Given inequalities are x + y ≤ 4, 2x + y ≤ 7.
Consider line L1 : x + y = 4 and L2 : 2x + y = 7
For line L1, A (0, 4) and B (4, 0)
For line L2, P(0, 7) and Q`(7/2, 0)`
Solving both lines, we get x = 3, y = 1.
The coordinates of origin O (0, 0) satisfies both the inequalities.
∴ The required region is on the origin side of both the lines L1 and L2.
As x ≥ 0, y ≥ 0, the feasible region is in the first quadrant.
OQRAO is the required feasible region.
∴ The corner points are O (0, 0), Q`(7/2, 0)`, R (3, 1), A (0, 4).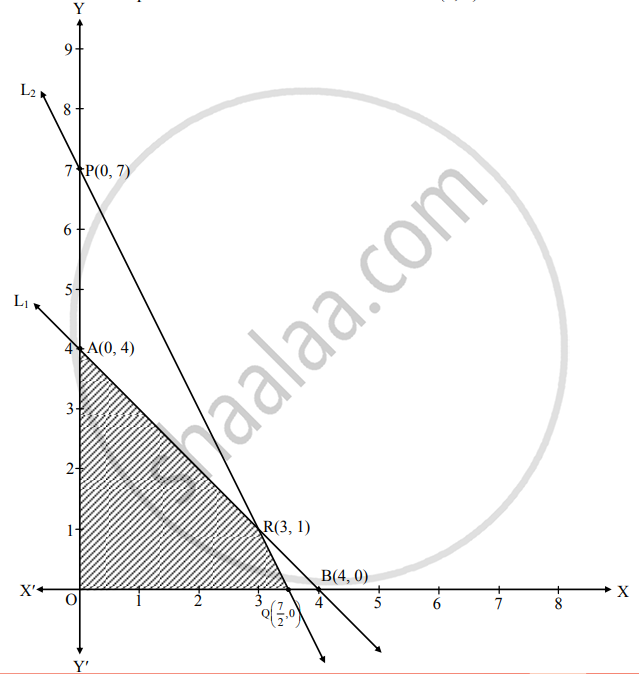
APPEARS IN
संबंधित प्रश्न
Which of the following statements is correct?
Find the feasible solution of the following inequations:
x - 2y ≤ 2, x + y ≥ 3, - 2x + y ≤ 4, x ≥ 0, y ≥ 0
A printing company prints two types of magazines A and B. The company earns ₹ 10 and ₹ 15 in magazines A and B per copy. These are processed on three Machines I, II, III. Magazine A requires 2 hours on Machine I, 5 hours on Machine II, and 2 hours on machine III. Magazine B requires 3 hours on machine I, 2 hours on machine II and 6 hours on Machine III. Machines I, II, III are available for 36, 50, and 60 hours per week respectively. Formulate the LPP to determine weekly production of magazines A and B, so that the total profit is maximum.
The corner points of the feasible solution are (0, 0), (2, 0), `(12/7, 3/7)`, (0, 1). Then z = 7x + y is maximum at ______.
If the corner points of the feasible solution are (0, 10), (2, 2) and (4, 0), then the point of minimum z = 3x + 2y is ______.
The half-plane represented by 3x + 2y < 8 contains the point ______.
A chemical company produces a chemical containing three basic elements A, B, C, so that it has at least 16 litres of A, 24 litres of B and 18 litres of C. This chemical is made by mixing two compounds I and II. Each unit of compound I has 4 litres of A, 12 litres of B and 2 litres of C. Each unit of compound II has 2 litres of A, 2 litres of B and 6 litres of C. The cost per unit of compound I is ₹ 800 and that of compound II is ₹ 640. Formulate the problems as LPP and solve it to minimize the cost.
A firm manufactures two products A and B on which profit earned per unit ₹ 3 and ₹ 4 respectively. Each product is processed on two machines M1 and M2. The product A requires one minute of processing time on M1 and two minutes of processing time on M2, B requires one minute of processing time on M1 and one minute of processing time on M2. Machine M1 is available for use for 450 minutes while M2 is available for 600 minutes during any working day. Find the number of units of product A and B to be manufactured to get the maximum profit.
Objective function of LPP is ______.
Which value of x is in the solution set of inequality − 2X + Y ≥ 17
Choose the correct alternative:
Z = 9x + 13y subjected to constraints 2x + 3y ≤ 18, 2x + y ≤ 10, 0 ≤ x, y was found to be maximum at the point
A company produces two types of products say type A and B. Profits on the two types of product are ₹ 30/- and ₹ 40/- per kg respectively. The data on resources required and availability of resources are given below.
| Requirements | Capacity available per month | ||
| Product A | Product B | ||
| Raw material (kgs) | 60 | 120 | 12000 |
| Machining hours/piece | 8 | 5 | 600 |
| Assembling (man hours) | 3 | 4 | 500 |
Formulate this problem as a linear programming problem to maximize the profit.
Solve the following linear programming problems by graphical method.
Minimize Z = 3x1 + 2x2 subject to the constraints 5x1 + x2 ≥ 10; x1 + x2 ≥ 6; x1 + 4x2 ≥ 12 and x1, x2 ≥ 0.
The values of θ satisfying sin7θ = sin4θ - sinθ and 0 < θ < `pi/2` are ______
The minimum value of z = 5x + 13y subject to constraints 2x + 3y ≤ 18, x + y ≥ 10, x ≥ 0, y ≥ 2 is ______
Solve the following LPP:
Maximize z = 7x + 11y, subject to 3x + 5y ≤ 26, 5x + 3y ≤ 30, x ≥ 0, y ≥ 0.
Food F1 contains 2, 6, 1 units and food F2 contains 1, 1, 3 units of proteins, carbohydrates, fats respectively per kg. 8, 12 and 9 units of proteins, carbohydrates and fats is the weekly minimum requirement for a person. The cost of food F1 is Rs. 85 and food F2 is Rs. 40 per kg. Formulate the L.P.P. to minimize the cost.
Sketch the graph of the following inequation in XOY co-ordinate system.
x + y ≤ 0
