Advertisements
Advertisements
प्रश्न
Construct the following and give justification:
A right triangle when one side is 3.5 cm and sum of other sides and the hypotenuse is 5.5 cm.
उत्तर
In the right triangle ABC, given BC = 3.5 cm, ∠B = 90° and sum of other side and hypotenuse be, AB + AC = 5.5 cm
To construct a triangle ABC use the following steps:
1. Draw the base BC = 3.5 cm
2. Make an angle XBC = 90° at the point B of base BC.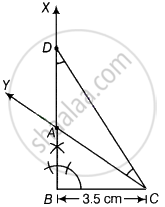
3. Cut the line segment BD equal to AB + AC i.e., 5.5 cm from the ray XB.
4. Join DC and make an ∠DCY equal to ∠BDC.
5. Let Y intersect BX at A. Therefore, ABC is the required triangle.
Justification:
Base BC and ∠B are drawn as given.
In ΔACD, ∠ACD = ∠ADC ...[By construction]
AD = AC ...(i) [Sides opposite to equal angles are equal]
Now, AB = BD – AD = BD – AC ...[From equation (i)]
BD = AB + AC
Hence, our construction is justified.
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC in which BC = 7 cm, ∠B = 75° and AB + AC = 13 cm.
Construct a triangle XYZ in which ∠Y = 30°, ∠Z = 90° and XY + YZ + ZX = 11 cm.
Using ruler and compasses only, construct a ΔABC, given base BC = 7cm, ∠ABC = 60° and AB + AC = 12 cm.
Construct a triangle whose perimeter is 6.4 cm, and angles at the base are 60° and 45° .
Construct a triangle ABC such that BC = 6 cm, AB = 6 cm and median AD = 4 cm.
With the help of a ruler and a compass it is not possible to construct an angle of ______.
An angle of 42.5° can be constructed.
A triangle ABC can be constructed in which AB = 5 cm, ∠A = 45° and BC + AC = 5 cm.
Construct a triangle whose sides are 3.6 cm, 3.0 cm and 4.8 cm. Bisect the smallest angle and measure each part.
Construct the following and give justification:
A rhombus whose diagonals are 4 cm and 6 cm in lengths.
