Advertisements
Advertisements
प्रश्न
Determine the volume contraction of a solid copper cube, 10 cm on an edge, when subjected to a hydraulic pressure of 7.0 ×106 Pa.
उत्तर १
Length of an edge of the solid copper cube, l = 10 cm = 0.1 m
Hydraulic pressure, p = 7.0 ×106 Pa
Bulk modulus of copper, B = 140 × 109 Pa
Bulk Modulus, `B = p/((triangle V)/V)`
Where
`(triangle V)/V` = Volumetric strain
ΔV = Change in volume
V = Original volume.
`triangleV = (pV)/B`
Original volume of the cube, `V = beta`
`:.triangle V = (pl^3)/B`
`= (7xx10^6xx (0.1)^3)/(140xx10^9)`
= 5 x 10-8 m3
= 5 x 10-2 cm-3
Therefore, the volume contraction of the solid copper cube is 5 × 10–2 cm–3.
उत्तर २
Here a side of copper cube a = 10 cm, hence volume V = a3 = 10-3 m3 , hydraulic pressure applied p = 7.0 x 106 Pa and from table we find that bulk modulus of copper B = 140 G Pa = 140 x 109 Pa.
Using the relation `B = p/((triangle V)/V)` we have decreased in
volume `triangle V = (PV)/B`
`:. triangleV = (7.0xx10^6xx10^(-3))/(140xx10^9) = 5xx 10^(-8) m^3 = 5 xx 10^(-2) cm^3`
APPEARS IN
संबंधित प्रश्न
A rigid bar of mass 15 kg is supported symmetrically by three wires each 2.0 m long. Those at each end are of copper and the middle one is of iron. Determine the ratio of their diameters if each is to have the same tension.
A mild steel wire of length 1.0 m and cross-sectional area 0.50 × 10–2 cm2 is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid-point of the wire. Calculate the depression at the midpoint.
A rope 1 cm in diameter breaks if the tension in it exceeds 500 N. The maximum tension that may be given to a similar rope of diameter 2 cm is
When a metal wire is stretched by a load, the fractional change in its volume ∆V/V is proportional to
Answer in one sentence.
How should be a force applied on a body to produce shearing stress?
Modulus of rigidity of ideal liquids is ______.
The maximum load a wire can withstand without breaking, when its length is reduced to half of its original length, will ______.
A rectangular frame is to be suspended symmetrically by two strings of equal length on two supports (Figure). It can be done in one of the following three ways;
| (a) | 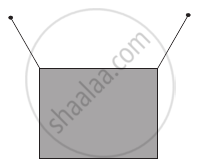 |
| (b) |  |
| (c) | 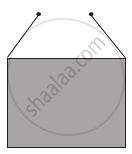 |
The tension in the strings will be ______.
The area of the cross-section of the rope used to lift a load by a crane is 2.5 × 10-4m2. The maximum lifting capacity of the crane is 10 metric tons. To increase the lifting capacity of the crane to 25 metric tons, the required area of cross-section of the rope should be ______.
(take g = 10 ms-2)
The stress-strain graph of a material is shown in the figure. The region in which the material is elastic is ______.

