Advertisements
Advertisements
प्रश्न
Draw a right triangle in which the sides (other than the hypotenuse) are of lengths 4 cm and 3 cm. Now construct another triangle whose sides are `3/5` times the corresponding sides of the given triangle.
उत्तर
Construct a right triangle of sides AB = 4 cm, AC = 3 cm and ∠A = 90° and then a triangle similar to it whose sides are `(3/5)^"th"` of the corresponding sides of ΔABC
We follow the following steps to construct the given
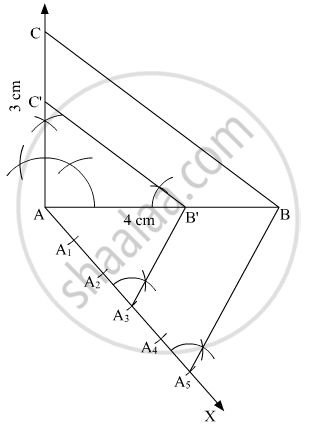
Step of construction
Step: I- First of all we draw a line segment AB = 4 cm.
Step: II- With A as centre and draw an angle ∠A = 90°.
Step: III- With A as centre and radius AC = 3 cm.
Step: IV-Join BC to obtain right ΔABC.
Step: V- Below AB, makes an acute angle ∠BAX.
Step: VI- Along AX, mark off five points A1, A2, A3, A4 and A5 such that =A4A5.
Step: VII- Join A5B.
Step: VIII -Since we have to construct a triangle each of whose sides is (`3/5`)th of the corresponding sides of right ΔABC
So, we draw a line on AX from point which is A3B ∥ A5B and meeting AB at B’.
Step: IX- From B’ point draw B'C' || BCand meeting AC at C’
Thus, ΔABC is the required triangle, each of whose sides is `(3/5)`th of the corresponding sides of ΔABC.
संबंधित प्रश्न
Write down the equation of a line whose slope is 3/2 and which passes through point P, where P divides the line segment AB joining A(-2, 6) and B(3, -4) in the ratio 2 : 3.
Construct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are `7/5` of the corresponding sides of the first triangle. Give the justification of the construction.
Draw a triangle ABC with side BC = 7 cm, ∠B = 45°, ∠A = 105°. Then, construct a triangle whose sides are `4/3 `times the corresponding side of ΔABC. Give the justification of the construction.
Draw a right triangle ABC in which AC = AB = 4.5 cm and ∠A = 90°. Draw a triangle similar to ΔABC with its sides equal to (5/4)th of the corresponding sides of ΔABC.
If A(–14, –10), B(6, –2) is given, find the coordinates of the points which divide segment AB into four equal parts.
ΔRHP ~ ΔNED, In ΔNED, NE = 7 cm, ∠D = 30°, ∠N = 20° and `"HP"/"ED" = 4/5`. Then construct ΔRHP and ΔNED
To divide a line segment PQ in the ratio 5 : 7, first a ray PX is drawn so that ∠QPX is an acute angle and then at equal distances points are marked on the ray PX such that the minimum number of these points is ______.
If the perpendicular distance between AP is given, which vertices of the similar triangle would you find first?
What is the ratio `(AC)/(BC)` for the following construction: A line segment AB is drawn. A single ray is extended from A and 12 arcs of equal lengths are cut, cutting the ray at A1, A2… A12.A line is drawn from A12 to B and a line parallel to A12B is drawn, passing through the point A6 and cutting AB at C.
Draw a parallelogram ABCD in which BC = 5 cm, AB = 3 cm and ∠ABC = 60°, divide it into triangles BCD and ABD by the diagonal BD. Construct the triangle BD' C' similar to ∆BDC with scale factor `4/3`. Draw the line segment D'A' parallel to DA where A' lies on extended side BA. Is A'BC'D' a parallelogram?
