Advertisements
Advertisements
प्रश्न
Explain in detail the kinetic interpretation of temperature.
Advertisements
उत्तर
To understand the microscopic origin of temperature in the same way, from pressure exerted by a gas,
P = `1/3 "N"/"V""m"bar("v"^2)`
PV = `1/3"Nm"bar("v"^2)` ............(1)
Comparing the equation (1) with the ideal gas equation PV = NkT,
NkT = `1/3"Nm"bar("v"^2)`
kT = `1/3"m"bar("v"^2)` .........(2)
Multiply the above equation by `3/2` on both sides,
`3/2"kT" = 1/2"m"bar("v"^2)` ...........(3)
R.H.S. of the equation (3) is called average kinetic energy of a single molecule `(bar"KE")`.
The average kinetic energy per molecule
`bar"KE"` = ∈ = `3/2"kT"` ...........(4)
Equation (3) implies that the temperature, of a gas, is a measure of the average translational kinetic energy per molecule of the gas.
Equation (4) is a very important result of the kinetic theory of gas. We can infer the following from this equation.
(i) The average kinetic energy of the molecule is directly proportional to the absolute temperature of the gas. Equation (3) gives the connection between the macroscopic world (temperature) to the microscopic world (motion of molecules).
(ii) The average kinetic energy of each molecule depends only on the temperature of the gas hot on the mass of the molecule. In other words, if the temperature of an ideal gas is measured using the thermometer, the average kinetic energy of each molecule can be calculated without seeing the molecule through the naked eye.
By multiplying the total number of gas molecules with the average kinetic energy of each molecule, the internal energy of the gas is obtained.
Internal energy of ideal gas U = `"N"(1/2"m"bar("v"^2))"`
By using equation (3), U = `3/2"NkT"` ..........(5)
Here, we understand that the internal energy of an ideal gas depends only on absolute temperature and is independent of pressure and volume.
APPEARS IN
संबंधित प्रश्न
Is it possible to boil water at room temperature, say 30°C? If we touch a flask containing water boiling at this temperature, will it be hot?
A gas cylinder has walls that can bear a maximum pressure of 1.0 × 106 Pa. It contains a gas at 8.0 × 105 Pa and 300 K. The cylinder is steadily heated. Neglecting any change in the volume, calculate the temperature at which the cylinder will break.
The weather report reads, "Temperature 20°C : Relative humidity 100%". What is the dew point?
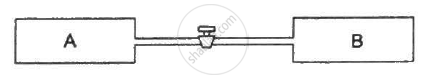
Figure shows two rigid vessels A and B, each of volume 200 cm3, containing an ideal gas (Cv = 12.5 J K−1 mol−1). The vessels are connected to a manometer tube containing mercury. The pressure in both the vessels is 75 cm of mercury and the temperature is 300 K. (a) Find the number of moles of the gas in each vessel. (b) 5.0 J of heat is supplied to the gas in vessel A and 10 J to the gas in vessel B. Assuming there's no appreciable transfer of heat from A to B, calculate the difference in the heights of mercury in the two sides of the manometer. Gas constant, R = 8.3 J K−1 mol−1.
Explain, on the basis of the kinetic theory of gases, how the pressure of a gas changes if its volume is reduced at a constant temperature.
When photons of energy hv fall on a metal plate of work function 'W0', photoelectrons of maximum kinetic energy 'K' are ejected. If the frequency of the radiation is doubled, the maximum kinetic energy of the ejected photoelectrons will be ______.
Average kinetic energy of H2 molecule at 300K is 'E'. At the same temperature, average kinetic energy of O2 molecule will be ______.
Assuming the expression for the pressure exerted by the gas on the wall of the container, it can be shown that pressure is ______.
A proton, a deuteron and an α-particle with same kinetic energy enter into a uniform magnetic field at right angle to magnetic field. The ratio of the radii of their respective circular paths is ______.
According to the kinetic theory of gases, at a given temperature, molecules of all gases have the same ______.
