Advertisements
Advertisements
प्रश्न
Find the average frictional force needed to stop a car weighing 500 kg at a distance of 25 m if the initial speed is 72 km/h.
उत्तर
\[\text{ Given } : \]
\[\text{ Mass of the car, m = 500 kg } \]
\[\text{ Distance covered by the car, s = 25 m }\]
\[\text{ Initial speed of the car, u = 72 km/h = 20 m/s } \]
\[\text{ Final speed of the car, } \nu = \text{ 0 m/s } \]
Retardation of the car,
\[a = \frac{\left( \nu^2 - u^2 \right)}{2s}\]
\[ \Rightarrow a = - \frac{400}{50} = - 8 \text{ m/ s}^2 \]
\[\text{ Frictional force, F = ma } = 500 \times 8 = 4000 N\]
APPEARS IN
संबंधित प्रश्न
List all the forces acting on the block B in figure.
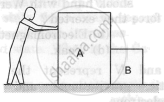
Figure shows a boy pulling a wagon on a road. List as many forces as you can which are relevant with this figure. Find the pairs of forces connected by Newton's third law of motion.
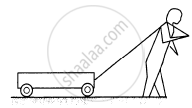
Figure shows a cart. Complete the table shown below.
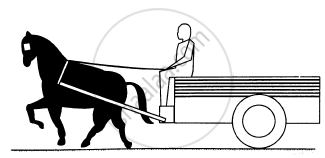
| Force on | Force by | Nature of the Force | Direction |
| Cart |
1 |
||
| Horse |
1 |
||
| Driver |
1 |
At what distance should two charges, each equal to 1 C, be placed so that the force between them equals your weight ?
The work done by all the forces (external and internal) on a system equals the change in ______.
A block of mass m slides down a smooth vertical circular track. During the motion, the block is in
No work is done by a force on an object if
(a) the force is always perpendicular to its velocity
(b) the force is always perpendicular to its acceleration
(c) the object is stationary but the point of application of the force moves on the object
(d) the object moves in such a way that the point of application of the force remains fixed.
A block of mass 5.0 kg slides down an incline of inclination 30° and length 10 m. Find the work done by the force of gravity.
A constant force of 2⋅5 N accelerates a stationary particle of mass 15 g through a displacement of 2⋅5 m. Find the work done and the average power delivered.
A block of mass m is kept over another block of mass M and the system rests on a horizontal surface (In the following figure). A constant horizontal force F acting on the lower block produces an acceleration \[\frac{F}{2 \left( m + M \right)}\] in the system, and the two blocks always move together. (a) Find the coefficient of kinetic friction between the bigger block and the horizontal surface. (b) Find the frictional force acting on the smaller block. (c) Find the work done by the force of friction on the smaller block by the bigger block during a displacement d of the system.

A block of weight 100 N is slowly moved up a smooth incline of inclination 37° by a person. Calculate the work done by the person in moving the block through a distance of 2 m, if the driving force is (a) parallel to the incline and (b) in the horizontal direction.
A 250 g block slides on a rough horizontal table. Find the work done by the frictional force in bringing the block to rest if it is initially moving at a speed of 40 cm/s. If the friction coefficient between the table and the block is 0⋅1, how far does the block move before coming to rest?
A uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is μ . Find the work done by friction during the period the chain slips off the table.
A force F = 20 + 10y acts on a particle in y-direction where F is in newton and y in metre. Work done by this force to move the particle from y – 0 to y – 1 m is:
A bicyclist comes to a skidding stop in 10 m. During this process, the force on the bicycle due to the road is 200 N and is directly opposed to the motion. The work done by the cycle on the road is ______.
A body of mass 0.5 kg travels in a straight line with velocity v = a x3/2 where a = 5 m–1/2s–1. The work done by the net force during its displacement from x = 0 to x = 2 m is ______.
A block of mass m is taken from A to B slowly under the action of a constant force R Work done by this force is ______.

Force acting on a particle is (2`hat"i"` + 3 `hat"j"`) N. Work done by this force is zero, when a particle is moved on the line 3y + kx = 5. Here value of k is ______.
