Advertisements
Advertisements
प्रश्न
Find the area of a triangle formed by the lines 3x + y – 2 = 0, 5x + 2y – 3 = 0 and 2x – y – 3 = 0
उत्तर
3x + y = 2 ...(1)
5x + 2y = 3 ...(2)
2x – y = 3 ...(3)
Solve (1) and (2) to get the vertices B
(1) × 2 ⇒ 6x + 2y = 4 ...(1)
(1) × 2 ⇒ 5x + 2y = 3 ...(2)
(−) (−) (−)
Subtract (1) and (2) ⇒ x = 1
Substitute the value of x = 1 in (1)
3(1) + y = 2
y = 2 – 3 = – 1
The point B is (1, – 1)
Solve (2) and (3) to get the vertices C
(2) × 1 ⇒ 5x + 2y = 3 ...(2)
(3) × 2 ⇒ 4x – 2y = 6 ...(4)
Adding (2) and (4) ⇒ 9x = 9
x = 1
Substitute the value of x = 1 in (3)
2(1) – y = 3
⇒ – y = 3 – 2
– y = 1
⇒ y = – 1
The point C is (1, – 1)
Solve (1) and (3) to get the vertices A
3x + y = 2 ...(1)
2x – 2y = 3 ...(2)
By adding (1) and (2) ⇒ 5x = 5
Substitute the value of x = 1 in (1)
3(1) + y = 2
y = 2 – 3 = – 1
The point A is (1, – 1)
The points A (1, – 1), B (1, – 1), C(1, – 1)
Area of ∆ABC = `1/2[x_1y_2 + x_2y_3 + x_3y_1 - (x_2y_1 + x_3y_2 + x_1y_3)]`
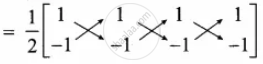
= `1/2 [-1 + (- 1)+ (- 1) - (- 1 + (- 1) + (- 1))]`
= `1/2[-3 - (- 3)]`
= `1/2[- 3 + 3]`
= `1/2 xx 0` = 0
Area of the triangle = 0 sq. units.
APPEARS IN
संबंधित प्रश्न
A rhombus sheet, whose perimeter is 32 m and whose one diagonal is 10 m long, is painted on both sides at the rate of Rs 5 per m2. Find the cost of painting.
Sides of a triangle are cm 45 cm, 39 cm and 42 cm, find its area.
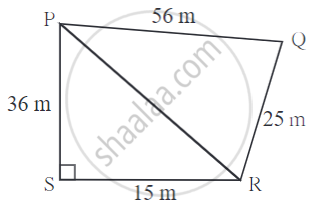
Look at the measures shown in the adjacent figure and find the area of ☐ PQRS.
Determine whether the sets of points are collinear?
`(-1/2, 3), (-5, 6) and (-8, 8)`
A man walks near a wall, such that the distance between him and the wall is 10 units. Consider the wall to be the Y-axis. The path travelled by the man is
The perimeter of a triangular plot is 600 m. If the sides are in the ratio 5 : 12 : 13, then find the area of the plot
Find the area of the unshaded region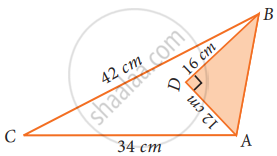
If the side of a rhombus is 10 cm and one diagonal is 16 cm, the area of the rhombus is 96 cm2.
From a point in the interior of an equilateral triangle, perpendiculars are drawn on the three sides. The lengths of the perpendiculars are 14 cm, 10 cm and 6 cm. Find the area of the triangle.
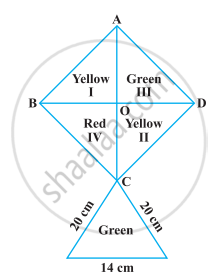
How much paper of each shade is needed to make a kite given in the following figure, in which ABCD is a square with diagonal 44 cm.