Advertisements
Advertisements
प्रश्न
From a point in the interior of an equilateral triangle, perpendiculars are drawn on the three sides. The lengths of the perpendiculars are 14 cm, 10 cm and 6 cm. Find the area of the triangle.
उत्तर
Let ABC be an equilateral triangle and O be the interior point and AQ, BR and CP are the perpendiculars drawn from point O such that OQ = 10 cm, OR = 6 cm, OP = 14 cm.
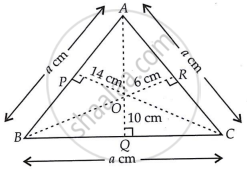
Let sides of an equilateral triangle be a cm.
Area of ΔOAB =
=
= 7a cm2 ...(i)
Area of ΔOBC =
=
= 5a cm2 ...(ii)
Area of ΔOAC =
= 3a cm2 ...(iii)
∴ Area of ΔABC = Area of (ΔOAB + ΔOBC + ΔOAC)
= (7a + 5a + 3a) cm2
= 15a cm2 ...(iv) [From (i), (ii) and (iii)]
Area of an equilateral ΔABC =
From (iv) and (v),
Substituting
Area of ΔABC =
=
Thus, the area of an equilateral triangle is
APPEARS IN
संबंधित प्रश्न
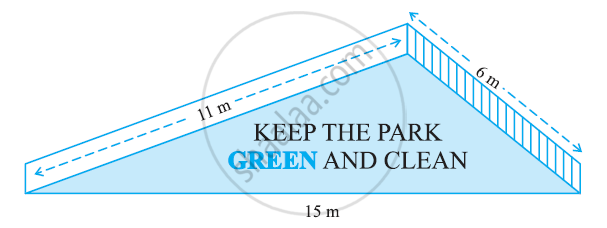
There is a slide in the park. One of its side walls has been painted in some colour with a message “KEEP THE PARK GREEN AND CLEAN” (see the given figure). If the sides of the wall are 15m, 11m, and 6m, find the area painted in colour.
An isosceles triangle has perimeter 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.
Find the area of a quadrilateral ABCD in which AB = 42 cm, BC = 21 cm, CD = 29 cm, DA =34 cm and diagonal BD =20 cm.
Find the areas of the given plot. (All measures are in metres.)
Determine whether the sets of points are collinear?
(a, b + c), (b, c + a) and (c, a + b)
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
A triangular shaped glass with vertices at A(– 5, – 4), B(1, 6) and C(7, – 4) has to be painted. If one bucket of paint covers 6 square feet, how many buckets of paint will be required to paint the whole glass, if only one coat of paint is applied
The perimeter of an equilateral triangle is 30 cm. The area is
The area of the isosceles triangle is
The cost of levelling the ground in the form of a triangle having the sides 51 m, 37 m and 20 m at the rate of Rs 3 per m2 is Rs 918.
