Advertisements
Advertisements
प्रश्न
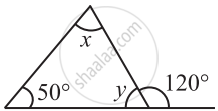
Find the value of the unknown x and y in the following diagram:

उत्तर
y = 90° (Vertically opposite angles)
x + x + y = 180° (Angle sum property)
2x + y = 180°
2x + 90° = 180°
2x = 180° − 90°
2x = 90°
x = `(90°)/2`
x = 45°
APPEARS IN
संबंधित प्रश्न
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

Find the value of the unknown x in the following diagram:

Find the value of the unknown x and y in the following diagram:
In ∆XYZ, if ∠X : ∠Z is 5 : 4 and ∠Y = 72°. Find ∠X and ∠Z
In ∆DEF, ∠F = 48°, ∠E = 68° and bisector of ∠D meets FE at G. Find ∠FGD
If one angle of a triangle is equal to the sum of the other two angles, then the triangle is ______.
It is possible to have a triangle in which each angle is greater than 60°.
In triangle XYZ, the measure of angle X is 30° greater than the measure of angle Y and angle Z is a right angle. Find the measure of ∠Y.
In ∆ABC, DE || BC (see figure). Find the values of x, y and z.

In the given figure, if ST = SU, then find the values of x and y.

