Advertisements
Advertisements
प्रश्न
In triangle XYZ, the measure of angle X is 30° greater than the measure of angle Y and angle Z is a right angle. Find the measure of ∠Y.
उत्तर
According to the question,
Measure of ∠X = ∠Y + 30°
Measure of ∠Z = 90°
We know that, the sum of all three angles in a triangle is equal to 180°
i.e. ∠X + ∠Y + ∠Z = 180°
⇒ ∠Y + (∠Y + 30°) + 90° = 180°
⇒ 2∠Y + 120° = 180°
⇒ 2∠Y = 180° – 120° = 60°
∴ ∠Y = `60^circ/2` = 30°
APPEARS IN
संबंधित प्रश्न
Find the value of the unknown x in the following diagram:

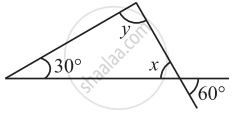
Find the value of the unknown x and y in the following diagram:
In the following triangle, find the value of x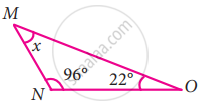
In ∆XYZ, if ∠X : ∠Z is 5 : 4 and ∠Y = 72°. Find ∠X and ∠Z
How many triangles can be drawn having its angles as 45°, 64° and 72°? Give reason for your answer.
Prove that a triangle must have atleast two acute angles.
In ∆ABC, ∠Α = 100°, AD bisects ∠A and AD ⊥ BC. Then, ∠B is equal to ______.
The sum of the measures of three angles of a triangle is greater than 180°.
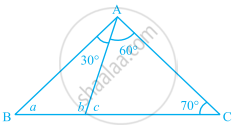
In the given figure, find the values of a, b and c.
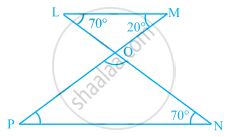
In the given figure, find the measures of ∠PON and ∠NPO.