Advertisements
Advertisements
Question
In triangle XYZ, the measure of angle X is 30° greater than the measure of angle Y and angle Z is a right angle. Find the measure of ∠Y.
Solution
According to the question,
Measure of ∠X = ∠Y + 30°
Measure of ∠Z = 90°
We know that, the sum of all three angles in a triangle is equal to 180°
i.e. ∠X + ∠Y + ∠Z = 180°
⇒ ∠Y + (∠Y + 30°) + 90° = 180°
⇒ 2∠Y + 120° = 180°
⇒ 2∠Y = 180° – 120° = 60°
∴ ∠Y = `60^circ/2` = 30°
APPEARS IN
RELATED QUESTIONS
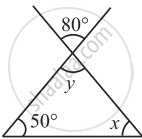
Find the value of the unknown x and y in the following diagram:
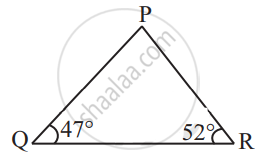
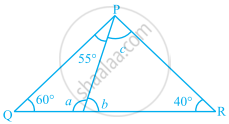
In the given figure find m∠P.
Two line segments `bar("AD")` and `bar("BC")` intersect at O. Joining `bar("AB")` and `bar("DC")` we get two triangles, ∆AOB and ∆DOC as shown in the figure. Find the ∠A and ∠B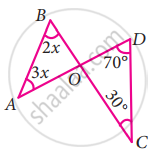
In a right angled triangle ABC, ∠B is right angle, ∠A is x + 1 and ∠C is 2x + 5. Find ∠A and ∠C
In a right angled triangle MNO, ∠N = 90°, MO is extended to P. If ∠NOP = 128°, find the other two angles of ∆MNO
In the given figure, which of the following statement is true?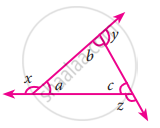
If one of the angles of a triangle is 110°, then the angle between the bisectors of the other two angles is ______.
In the given figure, find the values of a, b and c
The angles of a triangle are in the ratio 2:3:5. Find the angles.
In the given figure, if ST = SU, then find the values of x and y.

