Advertisements
Advertisements
प्रश्न
Find the type of the quadrilateral if points A(–4, –2), B(–3, –7) C(3, –2) and D(2, 3) are joined serially.
उत्तर
The given points are A(–4, –2), B(–3, –7) C(3, –2) and D(2, 3).
If they are joined serially so,
\[\text{Slope of AB} = \frac{y_{2}-y_{1}}{x_{2}-x_{1}}\]
= \[\frac{-7 - (-2)}{-3 - (-4)}\]
= \[\frac{- 7 + 2}{- 3 + 4} = - 5\]
\[\text{Slope of BC} = \frac{y_2-y_1}{x_2-x_1}\]
= \[\frac{-2-(-7)}{3-(-3)}\]
= \[\frac{- 2 + 7}{3 + 3} = \frac{5}{6}\]
\[\text{Slope of CD} = \frac{y_2-y_1}{x_2-x_1}\]
= \[\frac{3-(-2)}{2-3}\]
= \[\frac{3 + 2}{2 - 3} = - 5\]
\[\text{Slope of AD} = \frac{y_2-y_1}{x_2-x_1}\]
= \[\frac{3-(-2)}{2-(-4)}\]
= \[\frac{3 + 2}{2 + 4} = \frac{5}{6}\]
Slope of AB = slope of CD
∴ line AB || line CD
Slope of BC = slope of AD
∴ line BC || line AD
Both the pairs of opposite sides of ∆ABCD are parallel.
∴ ABCD is a parallelogram.
∴ The quadrilateral formed by joining the points A, B, C and D is a parallelogram.
APPEARS IN
संबंधित प्रश्न
Find the slope of the line passing through the points A(-2, 1) and B(0, 3).
Without using the distance formula, show that the points A(4, −2), B(−4, 4) and C(10, 6) are the vertices of a right-angled triangle.
Without using the distance formula, show that the points A(4, 5), B(1, 2), C(4, 3) and D(7, 6) are the vertices of a parallelogram.
The side AB of an equilateral triangle ABC is parallel to the x-axis. Find the slopes of all its sides.

The side AB of a square ABCD is parallel to the x-axis. Find the slopes of all its sides. Also, find:
- the slope of the diagonal AC.
- the slope of the diagonal BD.
The points (K, 3), (2, −4) and (−K + 1, −2) are collinear. Find K.
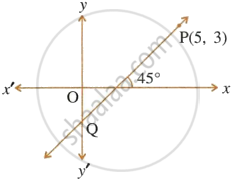
The line through P(5, 3) intersects y-axis at Q.
- Write the slope of the line.
- Write the equation of the line.
- Find the co-ordinates of Q.
Find the slope of the lines passing through the given point.
C (5, –2) , D (7, 3)
Determine whether the following point is collinear.
A(–4, 4), \[K\left( - 2, \frac{5}{2} \right),\] N (4, –2)
Fill in the blank using correct alternative.
Distance of point (–3, 4) from the origin is ______.
Show that points P(1, –2), Q(5, 2), R(3, –1), S(–1, –5) are the vertices of a parallelogram.
Find the slope of the diagonals of a quadrilateral with vertices A(1, 7), B(6, 3), C(0, –3) and D(–3, 3).
Find the slope of a line passing through the given pair of points (-5,-1) and (-9,-7)
Find the slope of a line parallel to the given line 5x + 2y = 11
Find the slope of a line passing through the points (x, 9) and (12, 6) is `(-1)/3 = ("y"_2 - "y"_1)/("x"_2 - "x"_1)`
Find the slope of the line passing through the points A(4,7) and B(2,3).
Find the Slope of the line having inclination 45°.
Find the value of x so that the line passing through (3, 4) and (x, 5) makes an angle 135° with positive direction of X-axis.
The line through A (- 2, 3) and B (4, b) is perpendicular to the line 2a – 4y = 5. Find the value of b.
What is the name of the point of intersection of coordinate axes?
