Advertisements
Advertisements
प्रश्न
From the given number line, find d(A, B):

उत्तर
Distance formula = (x2 – x1)
d(A, B) = 3 – (–3)
= 3 + 3
= 6 units.
APPEARS IN
संबंधित प्रश्न
Name the type of quadrilateral formed, if any, by the following points, and give reasons for your answer:
(- 1, - 2), (1, 0), (- 1, 2), (- 3, 0)
Find the distance between the following pair of points:
(a+b, b+c) and (a-b, c-b)
Find the values of x, y if the distances of the point (x, y) from (-3, 0) as well as from (3, 0) are 4.
Find the distance between the points
A(1,-3) and B(4,-6)
Find the distance between the points
P(a sin ∝,a cos ∝ )and Q( acos ∝ ,- asin ∝)
Find the distance of the following points from the origin:
(ii) B(-5,5)
Find the distances between the following point.
P(–6, –3), Q(–1, 9)
Find the distance of the following point from the origin :
(13 , 0)
Find the distance of a point (7 , 5) from another point on the x - axis whose abscissa is -5.
Find the coordinate of O , the centre of a circle passing through P (3 , 0), Q (2 , `sqrt 5`) and R (`-2 sqrt 2` , -1). Also find its radius.
The centre of a circle passing through P(8, 5) is (x+l , x-4). Find the coordinates of the centre if the diameter of the circle is 20 units.
P(5 , -8) , Q (2 , -9) and R(2 , 1) are the vertices of a triangle. Find tyhe circumcentre and the circumradius of the triangle.
A(2, 5), B(-2, 4) and C(-2, 6) are the vertices of a triangle ABC. Prove that ABC is an isosceles triangle.
Find the distance between the following pairs of points:
(-3, 6) and (2, -6)
A point P lies on the x-axis and another point Q lies on the y-axis.
If the abscissa of point P is -12 and the ordinate of point Q is -16; calculate the length of line segment PQ.
Prove that the points A (1, -3), B (-3, 0) and C (4, 1) are the vertices of an isosceles right-angled triangle. Find the area of the triangle.
The centre of a circle is (2x - 1, 3x + 1). Find x if the circle passes through (-3, -1) and the length of its diameter is 20 unit.
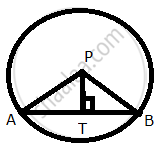
Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of AB.
Give the relation that must exist between x and y so that (x, y) is equidistant from (6, -1) and (2, 3).
The distance between point P(2, 2) and Q(5, x) is 5 cm, then the value of x ______
