Advertisements
Advertisements
प्रश्न
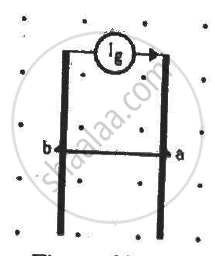
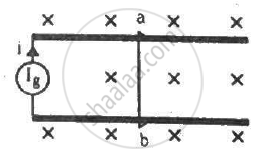
The current generator Ig' shown in figure, sends a constant current i through the circuit. The wire ab has a length l and mass m and can slide on the smooth, horizontal rails connected to Ig. The entire system lies in a vertical magnetic field B. The system is kept vertically in a uniform horizontal magnetic field B that is perpendicular to the plane of the rails (figure). It is found that the wire stays in equilibrium. If the wire ab is replaced by another wire of double its mass, how long will it take in falling through a distance equal to its length?
उत्तर
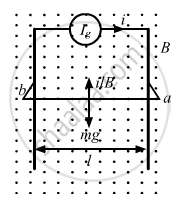
Let us consider the above free body diagram.
As the net force on the wire is zero, ilB = mg.
When the wire is replaced by a wire of double mass, we have
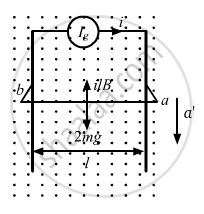
Now, let a' be the acceleration of the wire in downward direction and t be the time taken by the wire to fall.
Net force on the wire = 2mg − ilB = Fnet
On applying Newton's second law, we get
2mg − ilB = 2 ma' ...........(1)
\[\Rightarrow a' = \frac{2mg - ilB}{2 m}\]
\[s = ut + \frac{1}{2}a' t^2 \]
\[ \Rightarrow l = \frac{1}{2} \times \frac{2mg - ilB}{2m} \times t^2 .............\left[ \because s = l\right]\]
\[ \Rightarrow t = \sqrt{\frac{4 ml}{2mg - ilB}}\]
\[ \Rightarrow t = \sqrt{\frac{4 ml}{2mg - mg}} .........\text{[From (1)]}\]
` t = 2sqrt(l/g)`
APPEARS IN
संबंधित प्रश्न
Depict the behaviour of magnetic field lines in the presence of a diamagnetic material?
A point charge q moving with speed v enters a uniform magnetic field B that is acting into the plane of the paper as shown. What is the path followed by the charge q and in which plane does it move?
Two long straight parallel conductors carrying steady currents I1 and I2 are separated by a distance 'd'. Explain briefly, with the help of a suitable diagram, how the magnetic field due to one conductor acts on the other. Hence deduce the expression for the force acting between the two conductors. Mention the nature of this force.
A moving charge produces
Consider a long, straight wire of cross-sectional area A carrying a current i. Let there be n free electrons per unit volume. An observer places himself on a trolley moving in the direction opposite to the current with a speed \[v = \frac{i}{\text{nAe}}\] and separation from the wire by a distance r. The magnetic field seen by the observer is very nearly
The current generator Ig' shown in figure, sends a constant current i through the circuit. The wire ab has a length l and mass m and can slide on the smooth, horizontal rails connected to Ig. The entire system lies in a vertical magnetic field B. Find the velocity of the wire as a function of time.
A magnetic field that varies in magnitude from point to point but has a constant direction (east to west) is set up in a chamber. A charged particle enters the chamber and travels undeflected along a straight path with constant speed. What can you say about the initial velocity of the particle?
-
The presence of a large magnetic flux through a coil maintains a current in the coil if the circuit is continuous.
-
A coil of a metal wire kept stationary in a non– uniform magnetic field has an e.m.f induced in it.
-
A charged particle enters a region of uniform magnetic field at an angle of 85° to the magnetic lines of force, the path of the particle is a circle.
-
There is no change in the energy of a charged particle moving in a magnetic field although a magnetic force is acting on it.
A moving charge will gain kinetic energy due to the application of ______.
A charged particle moving in a magnetic field experiences a resultant force ______
A deuteron and an alpha particle having equal kinetic energy enter perpendicular into a magnetic field. Let `r_d` and `r_alpha` be their respective radii of the circular path. The value of `(r_d)/(r_alpha)` is equal to ______.
A beam of protons with speed 4 × 105 ms-1 enters a uniform magnetic field of 0.3 T at an angle of 60° to the magnetic field. The pitch of the resulting helical path of protons is close to :
(Mass of the proton = 1.67 × 10-27 kg, charge of the proton = 1.69 × 10-19 C)
A circular coil of radius 10 cm is placed in a uniform magnetic field of 3.0 × 10-5 T with its plane perpendicular to the field initially. It is rotated at constant angular speed about an axis along the diameter of coil and perpendicular to magnetic field so that it undergoes half of rotation in 0.2 s. The maximum value of EMF induced (in µV) in the coil will be close to the integer ______.
A thin strip 10 cm long is on a U-shaped wire of negligible resistance and it is connected to a spring of spring constant 0.5 Nm-1. The assembly is kept in a uniform magnetic field of 0.1 T. If the strip is pulled from its equilibrium position and released, the number of oscillations it performs before its amplitude decreases by a factor of e is N. If the mass of the strip is 50 grams, its resistance is 10 Ω, and air drag is negligible, N will be close to ______.

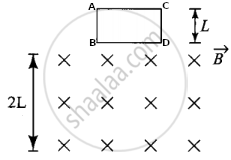
A square coil ABCD with its plane vertical is released from rest in a horizontal uniform magnetic field `vec"B"` of length 2L. The acceleration of the coil is ______.
A charge Q is moving `vec"dl"` distance in the magnetic field `vec"B"`. Find the value of work done by `vec"B"`.
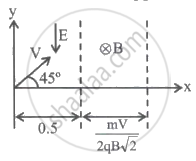
A charged particle of charge q and mass m is projected in a region that contains an electric and magnetic field as shown in the figure with velocity V at an angle of 45° with x-direction. If V = `sqrt((qE)/m)`, then net deviation in particle motion will be (neglect the effect of gravity) in a clockwise direction approx ______ °.