Advertisements
Advertisements
प्रश्न
If the angles of a triangle are in the ratio 1: 2 : 3, determine three angles.
उत्तर
Given that the angles of a triangle are in the ratio 1: 2 : 3
Let the angles be a, 2a,3a
∴ We know that
Sum of all angles of triangles is `180^@`
`a+2a+3a=180^@`
`6a=180^@`
`a=180^@/6`
`a=30^@`
Since` a=30^@`
`2a=2(30)^@=60^@`
`3a=3(30)^@=90^@`
∴ angles are a =` 30^@,2a=60^@,3a=90^@`
∴ Hence angles are `30^@,60^@ and 90^@`
APPEARS IN
संबंधित प्रश्न
The angles of a triangle are (x − 40)°, (x − 20)° and `(1/2x-10)^@.` find the value of x
Can a triangle have All angles less than 60° Justify your answer in case.
Fill in the blank to make the following statement true:
An exterior angle of a triangle is equal to the two ....... opposite angles.
Fill in the blank to make the following statement true:
A triangle cannot have more than ...... right angles.
An exterior angle of a triangle is 108° and its interior opposite angles are in the ratio 4 : 5. The angles of the triangle are
One angle of a triangle is 61° and the other two angles are in the ratio `1 1/2: 1 1/3`. Find these angles.
Find x, if the angles of a triangle is:
2x°, 4x°, 6x°
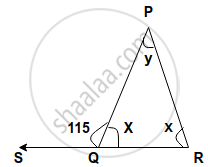
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
In a ∆ABC, AB = AC. The value of x is ________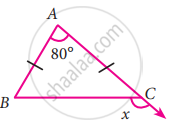
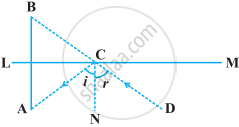
The image of an object placed at a point A before a plane mirror LM is seen at the point B by an observer at D as shown in the following figure. Prove that the image is as far behind the mirror as the object is in front of the mirror.
[Hint: CN is normal to the mirror. Also, angle of incidence = angle of reflection].