Advertisements
Advertisements
प्रश्न
If the bisector of the exterior vertical angle of a triangle be parallel to the base. Show that the triangle is isosce
उत्तर
Given that the bisector of the exterior vertical angle of a triangle is parallel to the base and we have to prove that the triangle is isosceles
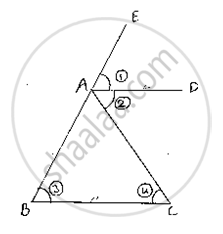
Let ABC be a triangle such that AD is the angular bisector of exterior vertical angle EAC and AD || BC
Let∠EAD = (1), ∠DAC = (2), ∠ABC = (3) and∠ACB = (4)
(1) = (2) [ ∵ AD is bisector of , ∠EAC ]
(1)=(3) [Corresponding angles]
and (2) = (4) [alternative angle]
⇒ (3) = (4) ⇒AB = AC
Since, in ΔABC, two sides AB and AC are equal we can say that
DABC is isosceles
APPEARS IN
संबंधित प्रश्न
In a ΔABC, if ∠A = 55°, ∠B = 40°, find ∠C.
Is the following statement true and false :
All the angles of a triangle can be greater than 60°.
Is the following statement true and false :
All the angles of a triangle can be equal to 60°.
Is the following statement true and false :
If one angle of a triangle is obtuse, then it cannot be a right angled triangle.
In ΔABC, if bisectors of ∠ABC and ∠ACB intersect at O at angle of 120°, then find the measure of ∠A.
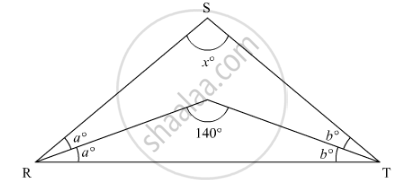
In ΔRST (See figure), what is the value of x?
Find x, if the angles of a triangle is:
x°, x°, x°
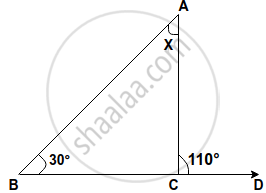
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
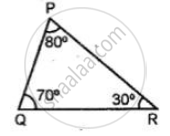
Classify the following triangle according to angle:
Can we have two acute angles whose sum is an acute angle? Why or why not?
