Advertisements
Advertisements
प्रश्न
If O is Any Point Inside a Rectangle Abcd Then `Oa^2+Oc^2=Ob^2+Od^2`
उत्तर
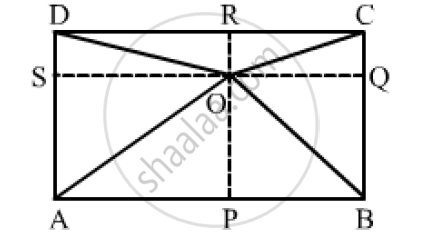
Suppose ABCD is a rectangle with O is any point inside it.
Construction: `OA^2+OC^2=OB^2+OD^2`
Proof:
`OA^2+OC^2=OB^2+OD^2` [Using Pythagoras theorem in right triangle AOP and COQ]
=`(BQ^2+OS^2)+(OQ^2+DS^2)`
=`(BQ^2+OQ^2)+(OS^2+DS^2)` [Using Pythagoras theorem in right triangle BOQ and DOS]
=`OB^2+OD^2`
Hence, LHS = RHS
APPEARS IN
संबंधित प्रश्न
All squares are ______.
State whether the following quadrilaterals are similar or not:

For each of the following statements state whether true(T) or false (F)
Two circles with different radii are similar.
For each of the following statements state whether true(T) or false (F)
The length of the line segment joining the midpoints of any two sides of a triangles is equal to half the length of the third side.
For each of the following statements state whether true(T) or false (F)
the polygon formed by joining the midpoints of the sides of a quadrilateral is a rhombus.
ΔABC is an equilateral A of side a. Its area will be ______.
The lengths of the diagonals of a rhombus are 24cm and 32cm, then the length of the altitude of the rhombus is ______.
Given below is the picture of the Olympic rings made by taking five congruent circles of radius 1cm each, intersecting in such a way that the chord formed by joining the point of intersection of two circles is also of length 1cm. Total area of all the dotted regions assuming the thickness of the rings to be negligible is ______.

The lengths of the diagonals of a rhombus are 16 cm and 12 cm. Then, the length of the side of the rhombus is ______.
Observe the figures given below carefully and answer the questions:
| Figure A | 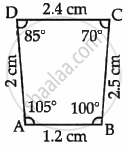 |
 |
| Figure B |  |
 |
| Figure C | 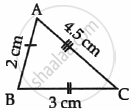 |
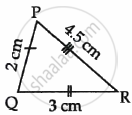 |
- Name the figure(s) where in two figures are similar.
- Name the figure(s) where in the figures are congruent.
-
- Prove that congruent triangles are also similar but not the converse.
OR - What more is least needed for two similar triangles to be congruent?
- Prove that congruent triangles are also similar but not the converse.
