Advertisements
Advertisements
प्रश्न
If O is Any Point Inside a Rectangle Abcd Then `Oa^2+Oc^2=Ob^2+Od^2`
उत्तर
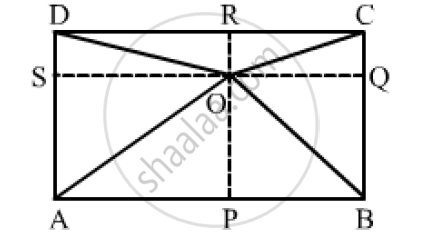
Suppose ABCD is a rectangle with O is any point inside it.
Construction: `OA^2+OC^2=OB^2+OD^2`
Proof:
`OA^2+OC^2=OB^2+OD^2` [Using Pythagoras theorem in right triangle AOP and COQ]
=`(BQ^2+OS^2)+(OQ^2+DS^2)`
=`(BQ^2+OQ^2)+(OS^2+DS^2)` [Using Pythagoras theorem in right triangle BOQ and DOS]
=`OB^2+OD^2`
Hence, LHS = RHS
APPEARS IN
संबंधित प्रश्न
State whether the following quadrilaterals are similar or not:

Write the truth value (T/F) of each of the following statement
Two polygons are similar, if their corresponding sides are proportional.
Write the truth value (T/F) of each of the following statement
Two triangles are similar if their corresponding angles are proportional.
For each of the following statements state whether true(T) or false (F)
Two circles with different radii are similar.
For each of the following statements state whether true(T) or false (F)
if two triangles are similar then their corresponding angles are equal and their corresponding sides are equal
For each of the following statements state whether true(T) or false (F)
The length of the line segment joining the midpoints of any two sides of a triangles is equal to half the length of the third side.
For each of the following statements state whether true(T) or false (F)
the polygon formed by joining the midpoints of the sides of a quadrilateral is a rhombus.
For each of the following statements state whether true(T) or false (F)
the ratio of the perimeter of two similar triangles is the same as the ratio of the their corresponding medians.
The symbol ~ is used to represent _________ triangles
In Figure, ∠BAC = 90° and AD ⊥ BC. Then, ______.