Advertisements
Advertisements
Question
If O is Any Point Inside a Rectangle Abcd Then `Oa^2+Oc^2=Ob^2+Od^2`
Solution
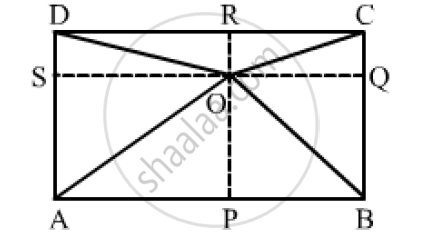
Suppose ABCD is a rectangle with O is any point inside it.
Construction: `OA^2+OC^2=OB^2+OD^2`
Proof:
`OA^2+OC^2=OB^2+OD^2` [Using Pythagoras theorem in right triangle AOP and COQ]
=`(BQ^2+OS^2)+(OQ^2+DS^2)`
=`(BQ^2+OQ^2)+(OS^2+DS^2)` [Using Pythagoras theorem in right triangle BOQ and DOS]
=`OB^2+OD^2`
Hence, LHS = RHS
APPEARS IN
RELATED QUESTIONS
Two triangles are similar, if their corresponding sides are .......... (proportional, equal)
Write the truth value (T/F) of each of the following statement
Any two congruent figures are similar.
For each of the following statements state whether true(T) or false (F)
if two triangles are similar then their corresponding angles are equal and their corresponding sides are equal
For each of the following statements state whether true(T) or false (F)
In a ΔABC , AB = 6 cm, ∠A = 45° and AC = 8 cm and in a ΔDEF , DF = 9 cm ∠D = 45° and DE= 12 cm then ΔABC ∼ΔDEF
For each of the following statements state whether true(T) or false (F)
the polygon formed by joining the midpoints of the sides of a quadrilateral is a rhombus.
For each of the following statements state whether true(T) or false (F)
The sum of the squares on the sides of a rhombus is equal to the sum of the squares on its diagonals.
The symbol ~ is used to represent _________ triangles
The height of a tower is measured by a mirror on the ground at R by which the top of the tower’s reflection is seen. Find the height of the tower. If ∆PQR ~ ∆STR

The lengths of the diagonals of a rhombus are 16 cm and 12 cm. Then, the length of the side of the rhombus is ______.
Observe the figures given below carefully and answer the questions:
| Figure A | 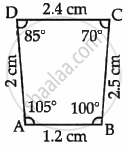 |
 |
| Figure B |  |
 |
| Figure C | 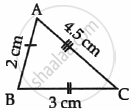 |
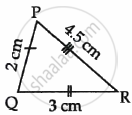 |
- Name the figure(s) where in two figures are similar.
- Name the figure(s) where in the figures are congruent.
-
- Prove that congruent triangles are also similar but not the converse.
OR - What more is least needed for two similar triangles to be congruent?
- Prove that congruent triangles are also similar but not the converse.
