Advertisements
Advertisements
प्रश्न
If we join a vertex to a point on opposite side which divides that side in the ratio 1:1, then what is the special name of that line segment?
पर्याय
Median
Angle bisector
Altitude
Hypotenuse
उत्तर
Median
Explanation:
Consider ΔABC in which AD divides BC in the ratio 1:1.
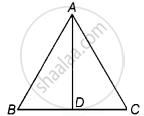
Now, BD:DC = 1:1
⇒ `(BD)/(DC) = 1/1`
∴ BD = DC
Since, AD divides BC into two equal parts.
Hence, AD is the median.
APPEARS IN
संबंधित प्रश्न
In ΔPQR, ∠Q = 90°, PQ = 12, QR = 5 and QS is a median. Find l(QS).
In the given figure, point G is the point of concurrence of the medians of Δ PQR. If GT = 2.5, find the lengths of PG and PT.

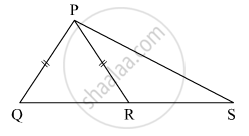
In the given figure, if seg PR ≅ seg PQ, show that seg PS > seg PQ.
Draw an obtuse angled Δ LMN. Draw its altitudes and denote the orthocentre by ‘O’.
Draw an isosceles triangle. Draw all of its medians and altitudes. Write your observation about their points of concurrence.
Identify the centroid of ∆PQR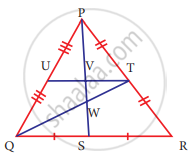
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If DE = 44, then DM = ?
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If PD = 12, then PN = ?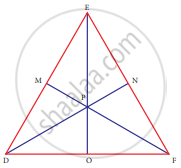
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If DO = 8, then FD = ?
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If OE = 36 then EP = ?
