Advertisements
Advertisements
प्रश्न
In a right-angled triangle, altitude is 2 cm longer than its base. Find the dimensions of the right-angled triangle given that the length of its hypotenuse is 10 cm.
उत्तर
Let x cm be the base of the right-angled triangle.
As a result, the altitudeude = (x + 2) cm.
Also, hypotenuse = 10 cm
We have the Pythagorean theorem.
(Base)2 + (Altitude)2 = (Hypotenuse)2
⇒ x2 + (x + 2)2 = 102
⇒ x2 + x2 + 4x + 4 = 100
⇒ 2x2 + 4x – 96 = 0
⇒ 2x + 2x – 48 = 0
⇒ x2 + (8 – 6)x – 48 = 0
⇒ x2 + 8x – 6x – 48 = 0
⇒ x(x + 8) – 6(x + 8) = 0
⇒ (x – 6)(x + 8) = 0
⇒ (x + 8) = 0 or (x – 6) = 0
⇒ x = – 8 or x = 6
A triangle's sides cannot be negative.
So, x = 6.
As a result, the right-angled triangle's base is 6 cm and its altitude is 6 + 2 = 8 cm.
As a result, the right-angled triangle's dimensions are 8 cm, 6 cm, and 10 cm.
APPEARS IN
संबंधित प्रश्न
If α and β are the roots of the equation `3x^2+8x+2=0` then (1/α+1/β)=?
(a)` -3/8` (b) `2/3` `(c) -4 (d)4`
If the equation `9x^26kx+4=0` has equal roots then k =?
(a)1 or (b)-1 or 4 (c)1 or -4 (d)-1 or -4
The length of a rectangular field exceeds its breadth by 8 m and the area of the field is `240 m^2` . The breadth of the field is
(a) 20 m (b) 30 m (c) 12 m (d) 16 m
The roots of the quadratic equation `2x^2-x-6=0`
(a)`-2, 3/2` (b) `2, -3/2`
(c)` -2, 3/2` (d) `2, 3/2`
Show that x= -2 is a solution of `3x^2+13x+14=0`
Solve` 2x^2+ax-a^2=0`
Solve `x^2+6x-(a^2+2a-8)=0`
If 1 is a root of the equations ay2 + ay + 3 = 0 and y2 + y + b = 0 then ab equals: ?
Solve for x: `3x^2-2sqrt6x+2=0`
In the orange garden of Mr. Madhusudan there are 150 orange trees. The number of trees in each row is 5 more than that in each column. Find the number of trees in each row and each column with the help of following flow chart.

is the following equation quadratic?
\[\left( x + 2 \right)^2 = 2 x^2\]
Solve the following quadratic equation.
\[x^2 - \frac{3x}{10} - \frac{1}{10} = 0\]
Alka spends 90 % of the amount sent to her and saves Rs. 120 per month. Find the amount sent to her per month.
Solve any four of the following.
Find the value of y in the equation x + y = 12, when x = 5
Two water taps together can fill a tank in `1 7/8` hours. The tap with a longer diameter takes 2 hours less than the tap with a smaller one to fill the tank separately. Find the time in which each tap can fill the tank separately.
A boat goes 30 km upstream and 44 km downstream in 10 hours. In 13 hours, it can go 40 km upstream and 55 km downstream. Determine the speed of the stream and that of the boat in still water.
For the quadratic equation 3x2 + 2x - 1 = 0 , find the value of the discriminant.
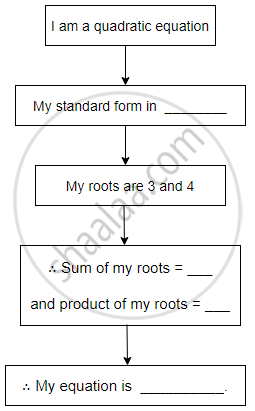
Complete the following activity to form a quadratic equation.
Activity:

In the adjoining fig. `square` ABCD is a trapezium AB || CD and its area is 33 cm2. From the information given in the figure find the lengths of all sides of the `square` ABCD. Fill in the empty boxes to get the solution.
Solution: `square` ABCD is a trapezium.
AB || CD
`"A"(square "ABCD") = 1/2 ("AB" + "CD") xx`______
33 = `1/2 ("x" + 2"x" + 1) xx `______
∴ ______ = (3x + 1) × ______
∴ 3x2 +______ − ______ = 0
∴ 3x(______) + 10(______) = 0
∴ (3x + 10) (______) = 0
∴ (3x + 10) = 0 or ______ = 0
∴ x = `-10/3` or x = ______
But length is never negative.
∴ `"x" ≠ -10/3`
∴ x = ______
AB = ______, CD = ______, AD = BC = ______
