Question

In a ΔABC if D and E are mid-points of BC and AD respectively such that ar (ΔAEC) = 4cm², then ar (ΔBEC) =

पर्याय

• 4 cm²

• 6 cm²

•  8 cm²

• 12 cm²

Answer 1

Given: In ΔABC

(1) D is the midpoint of BC

(2) E is the midpoint of AD

(3) ar (ΔAEC) = 4 cm²

To find: ar (ΔBEC)

Calculation: We know that”the median of the triangle divides the triangle into two triangle of equal area” 

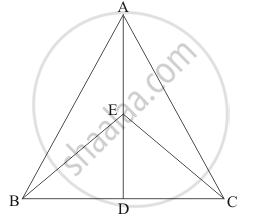
Since AD is the median of ΔABC,

ar (ΔABD) = ar (ΔADC) …… (1)

EC is the median of ΔADC,

ar (ΔAEC) = ar (ΔDEC) …… (2)

⇒ ar (ΔDEC) = 4 cm²

EC is the median of ΔBED

ar (ΔBED) = ar (ΔDEC) …… (3)

From 2 and 3 we get,

ar (ΔBED) = ar (ΔAEC) …… (4)

⇒ ar (ΔBED) = 4 cm²

Now,

ar (ΔBEC) = ar (ΔBED) + ar (ΔDEC)

                = 4 + 4 (subsituting the values)

ar(ΔBEC) = 8 cm²