Advertisements
Advertisements
प्रश्न
In fig. the centre of the circle is O. PQ and RS are two equal chords of the circle which , when produced , meet at T outside the circle . Prove that (a) TP = TR (b) TQ = TS.

उत्तर
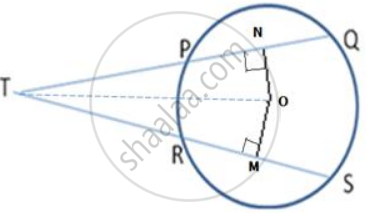
Given PQ = RS
To prove : TP = TR and TQ = TS
Construction : Draw ON ⊥ PQ and OM ⊥ RS
Proof : Since equal vhords are equidistance from the circle therefore
PQ = RS ⇒ ON = OM ....(1)
Also perpendicular drawn from the centre bisects the chord.
So, PN = NQ = `1/2` "PQ" and RM = MS = `1/2` "RS"
But PQ = RS , we get
PN = RM ......(2)
And , NQ = MS ....(3)
Now in Δ TMO and Δ TNO ,
TO = TO .....(common)
MO = NO .....(BY (1))
∠ TMO = ∠ TNO ...(Each 90 degrees)
Therefore , Δ TMO ≅ Δ TNO , ..(By RHS)
⇒ TN = TM ...(by CPCT) ...(4)
Substracting ,(2) from (4) , we get
TN - PN = TM - RM
⇒ TP = TR
Adding (3) and (4) , we get
TN + NQ = TM + MS
⇒ TQ = TS
Adding (3) and (4) , we get
TN + NQ = TM + MS
⇒ TQ = TS
Hence proved.
APPEARS IN
संबंधित प्रश्न
M and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. prove that:
(i) ∠BMN = ∠DNM.
(ii) ∠AMN = ∠CNM.
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the radius of the circle is 10 cm, find the area of the rhombus.
In a circle, with centre O, a cyclic quadrilateral ABCD is drawn with AB as a diameter of the circle and CD equal to radius of the circle. If AD and BC produced meet at point P; show that ∠APB = 60°.
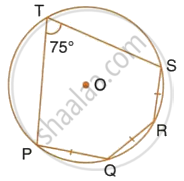
The given figure shows a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
- ∠POS,
- ∠QOR,
- ∠PQR.
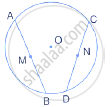
In fig, AB and CD are two equal chords of a circle with centre O. If M and N are the midpoints of AB and CD respectively,
prove that (a) ∠ ONM = ∠ ONM (b) ∠ AMN = ∠ CNM.
AB and AC are two equal chords of a circle with centre o such that LABO and LCBO are equal. Prove that AB = BC.
In fig., chords AB and CD of a circle intersect at P. AP = 5cm, BP= 3cm and CP = 2.5cm. Determine the length of DP.
Prove that equal chords of congruent circles subtend equal angles at their center.
Two equal circles intersect in P and Q. A straight line through P meets the circles in A and B. Prove that QA = QB
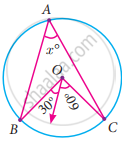
Find the value of x° in the following figure: