Advertisements
Advertisements
प्रश्न
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the radius of the circle is 10 cm, find the area of the rhombus.
उत्तर
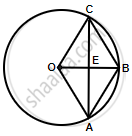
Radius = 10 cm
In rhombus OABC,
OC = 10 cm
∴ `OE = 1/2 xx OB`
= `1/2 xx 10`
= 5 cm
In right ΔOCE,
OC2 = OE2 + EC2
`=>` 102 = 52 + EC2
`=>` EC2 = 100 – 25 = 75
`=>` EC = `5sqrt(3)`
∴ AC = 2 × EC
= `2 xx 5sqrt(3)`
= `10sqrt(3)`
Area of rhombus = `1/2 xx OB xx AC`
= `1/2 xx 10 xx 10sqrt(3)`
= `50 sqrt(3) cm^2 ≈ 86.6 cm^2` ...`(sqrt(3) = 1.73)`
APPEARS IN
संबंधित प्रश्न
OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the area of the rhombus is `32sqrt(3) cm^2` find the radius of the circle.
In a circle, with centre O, a cyclic quadrilateral ABCD is drawn with AB as a diameter of the circle and CD equal to radius of the circle. If AD and BC produced meet at point P; show that ∠APB = 60°.
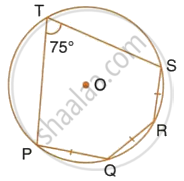
The given figure shows a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
- ∠POS,
- ∠QOR,
- ∠PQR.
Two congruent drdes have their centres at 0 and P. Mis the midpoint of the line segment OP. A straight line is drawn through M cutting the two circles at the points A, B, C and D. Prove that the chords AB and CD are equal.
In following figure .,XY and YZ are two equal chords of a circle with centre O. Prove that the bisector of ∠ XYZ passes through O.
AB and AC are two equal chords of a circle with centre o such that LABO and LCBO are equal. Prove that AB = BC.
In fig., chords AB and CD of a circle intersect at P. AP = 5cm, BP= 3cm and CP = 2.5cm. Determine the length of DP.
Two equal chords AB and CD of a circle with center O, intersect each other at point P inside the circle.
Prove that: (i) AP = CP ; (ii) BP = DP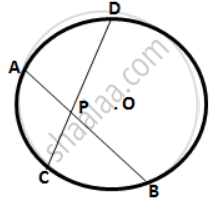
Prove that equal chords of congruent circles subtend equal angles at their center.
Two equal circles intersect in P and Q. A straight line through P meets the circles in A and B. Prove that QA = QB
