Advertisements
Advertisements
प्रश्न
AB and CD are two equal chords of a drde intersecting at Pas shown in fig. P is joined to O , the centre of the cirde. Prove that OP bisects ∠ CPB.
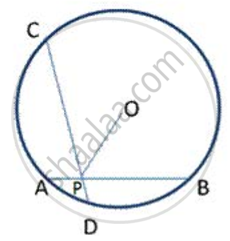
उत्तर

Draw perpendiculars OR and OS to CD and AB respectively.
In triangle ORP and triangle OSP
OP= OP
OR = OS (Distance of equal chords from centre are equal)
∠ PRO = ∠ PSO (right angles)
Therefore, Δ ORP ≅ Δ OSP
Hence, ∠ RPO = ∠ SPO
Thus OP bisects ∠ CPB.
APPEARS IN
संबंधित प्रश्न
In the given figure, the incircle of ∆ABC touches the sides BC, CA and AB at D, E, F respectively. Prove that AF + BD + CE = AE + CD + BF = `\frac { 1 }{ 2 } ("perimeter of ∆ABC")`
In the given figure, PQ and RS are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersects PQ at A and RS at B. Prove that ∠AOB = 90º
In fig. XP and XQ are tangents from X to the circle with centre O. R is a point on the circle. Prove that, XA + AR = XB + BR.
Fill in the blanks:
The centre of a circle lies in ____________ of the circle.
In the following figure, AB is the diameter of a circle with centre O and CD is the chord with length equal to radius OA.
Is AC produced and BD produced meet at point P; show that ∠APB = 60°
The figure given below shows a circle with center O in which diameter AB bisects the chord CD at point E. If CE = ED = 8 cm and EB = 4 cm,
find the radius of the circle.

In the above figure, seg AB is a diameter of a circle with centre P. C is any point on the circle. seg CE ⊥ seg AB. Prove that CE is the geometric mean of AE and EB. Write the proof with the help of the following steps:
a. Draw ray CE. It intersects the circle at D.
b. Show that CE = ED.
c. Write the result using the theorem of the intersection of chords inside a circle. d. Using CE = ED, complete the proof.
Use the figure given below to fill in the blank:
________ is a radius of the circle.
In the following figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to ______.

On a common hypotenuse AB, two right triangles ACB and ADB are situated on opposite sides. Prove that ∠BAC = ∠BDC.
