Advertisements
Advertisements
प्रश्न
In the following figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to ______.

पर्याय
30º
60º
90º
45º
उत्तर
In the following figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to 45º.
Explanation:
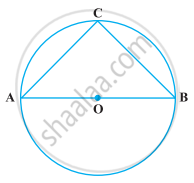
We know that, diameter subtends a right angle to the circle.
∴ ∠BCA = 90º ...(i)
Given, AC = BC
⇒ ∠ABC = ∠CAB ...(ii) [Angles opposite to equal sides are equal]
In ΔABC, ∠CAB + ∠ABC + ∠BCA = 180º ...[By angle sum property of a triangle]
⇒ ∠CAB + ∠CAB + ∠90º = 180º ...[From equations (i) and (ii)]
⇒ 2∠CAB = 180º – 90º
⇒ ∠CAB = `(90^circ)/2`
∴ ∠CAB = 45º
APPEARS IN
संबंधित प्रश्न
Prove that the line segment joining the points of contact of two parallel tangents of a circle, passes through its centre.
In the given figure, the incircle of ∆ABC touches the sides BC, CA and AB at D, E, F respectively. Prove that AF + BD + CE = AE + CD + BF = `\frac { 1 }{ 2 } ("perimeter of ∆ABC")`
From an external point P, tangents PA and PB are drawn to the circle with centre O. If CD is the tangent to the circle at point E and PA = 14 cm. Find the perimeter of ABCD.
In fig.. O is the center of the circle and BCD is tangent to it at C. Prove that ∠BAC +
∠ACD = 90°
A triangle PQR is drawn to circumscribe a circle of radius 8 cm such that the segments QT and TR, into which QR is divided by the point of contact T, are of lengths 14 cm and 16 cm respectively. If area of ∆PQR is 336 cm2, find the sides PQ and PR.
In Fig. 8.78, there are two concentric circles with centre O. PRT and PQS are tangents to the inner circle from a point P lying on the outer circle. If PR = 5 cm, find the length of PS.

ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.

The ratio between the circumference and diameter of any circle is _______
Find the missing values in the following table for the circles with radius (r), diameter (d) and Circumference (C).
| radius (r) | diameter (d) | Circumference (C) |
| 24 m |
From the figure, identify a diameter.

