Advertisements
Advertisements
प्रश्न
From an external point P, tangents PA and PB are drawn to the circle with centre O. If CD is the tangent to the circle at point E and PA = 14 cm. Find the perimeter of ABCD.
उत्तर
PA = 14 cm
Perimeter of ΔPCD = PC + PD + CD = PC + PD + CE + ED
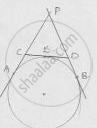
We know that
The two tangents drawn from external point to the circle are equal in length.
From point P, PA = PB = 14cm
From point C, CE = CA
From point D, DB = ED
Perimeter = PC + PD + CA +DB
= (PC + CA) + (PD + DB)
= PA + PB = 14 + 14 = 28 cm.
APPEARS IN
संबंधित प्रश्न
A chord PQ of a circle of radius 10 cm substends an angle of 60° at the centre of circle. Find the area of major and minor segments of the circle.
If \[d_1 , d_2 ( d_2 > d_1 )\] be the diameters of two concentric circle s and c be the length of a chord of a circle which is tangent to the other circle , prove that\[{d_2}^2 = c^2 + {d_1}^2\].
In Fig. 8.78, there are two concentric circles with centre O. PRT and PQS are tangents to the inner circle from a point P lying on the outer circle. If PR = 5 cm, find the length of PS.

Use the figure given below to fill in the blank:
Tangent to a circle is _______.

Mark two points A and B ,4cm a part, Draw a circle passing through B and with A as a center

Can the length of a chord of a circle be greater than its diameter ? Explain.
State, if the following statement is true or false:
If the end points A and B of the line segment lie on the circumference of a circle, AB is a diameter.
State, if the following statement is true or false:
The diameters of a circle always pass through the same point in the circle.
Twice the radius is ________________
Two circles with centres O and O' of radii 3 cm and 4 cm, respectively intersect at two points P and Q such that OP and O'P are tangents to the two circles. Find the length of the common chord PQ.
